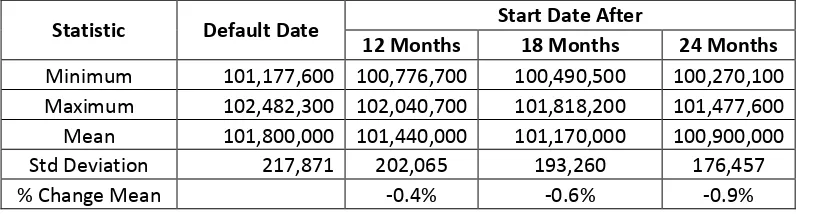
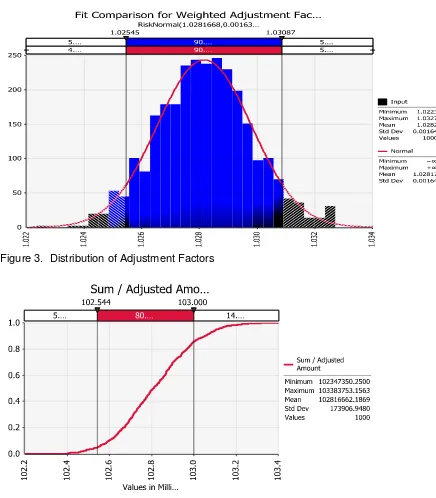
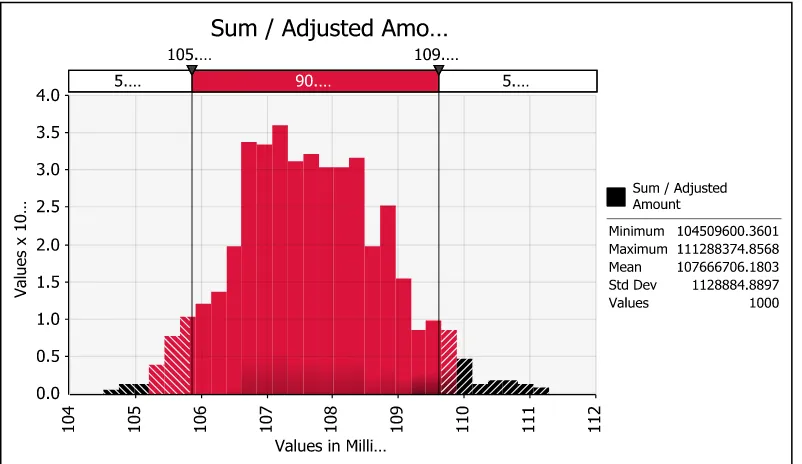
Costs and Benefits of the Price Adjustme
Teks penuh
Gambar
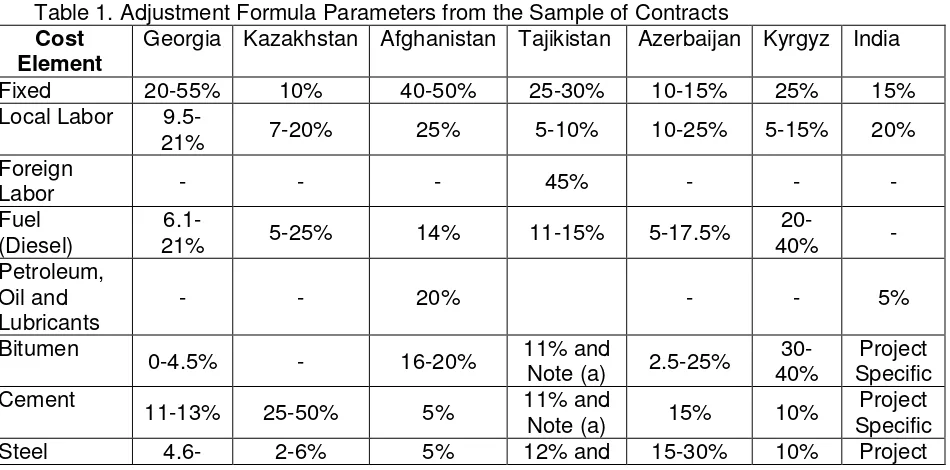
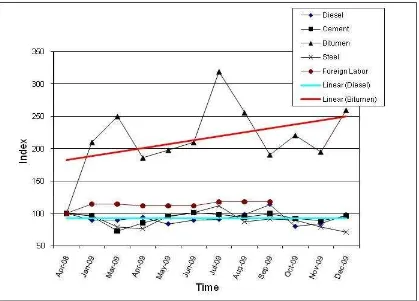
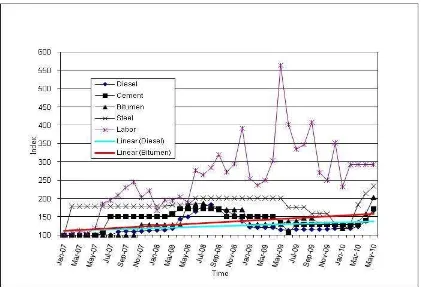
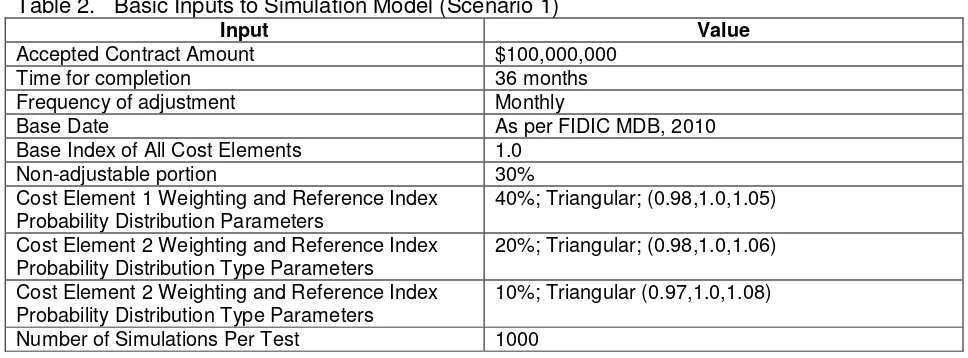
Garis besar
Dokumen terkait
[r]
Berdasarkan Surat Penetapan pemenang Lelang Nomor : 02/BAPPL/PAN-PERINDAG/2013 tanggal 25 Juni 2013, Perihal Penetapan pemenang Pelelangan Pengadaan Barang dan Jasa untuk
Peternakan Batu selaku Kuasa Pengguna Anggaran Nomor: 383/Kpts/PL.200/J3.6/05/2015 tanggal 26 Mei 2015, mengadakan Pemberian Penjelasan Pekerjaan (Aanwijzing) secara
Debu vulkanik gunung Sinabung memiliki struktur kristal yang secara mikroskopis terlihat tajam-tajam dipinggir seperti pecahan kaca yang tidak beraturan hal ini yang
In response to the question asked to determine the types of museum which the parents had visited with their children, a considerable number of the participants stated that they had
[r]
Mudharabah menurut ilmu Fiqh Syafi’iyah adalah pemberian modal dari pemilik dana kepada pengelola dana dengan tujuan agar dijalankan suatu usaha karena keuntungan yang dapat
Negara Republik Indonesia melakukan penambahan penyertaan modal negara ke dalam modal Perusahaan Umum (Perum) Sarana Pengembangan Usaha yang didirikan berdasarkan Peraturan