Arif Hossain, Department of Mathematics Khulna University of Engineering & Technology (KUET), Bangladesh for his encouragement and constructive suggestion. In this thesis, we have given some results on (relatively) pseudocomplemented lattices which certainly extended and generalized many results in lattice theory. We have considered this section as the basis and background for the study of subsequent sections.
For the background material in Lattice theory we have referred the readers to that of G. Then we showed that a distributive Lattice L with 0 is generalized stone if and only if it is both normal and sexually quasi-complemented. In chapter four we studied lattices with the largest element 1 where an antitone bijection is defined on each interval [a,1].
We have characterized these lattices using two induced binary operations that prove that the resulting algebras form a manifold. We have introduced a further generalization of this concept that defines the notion of a grating with sectional antitone bijection.
Preliminaries
Power set: The family of all subsets of a set is called the power set of X. Function: Let A and B be two sets, a relation R: A -p B is called a function if each element of A is mapped to a unique element of B. Domain and Co-domain: If the relation R A -* B is a function, set A is called domain and set B is called co-domain.
Then f is said to be a one-to-one function if every element of A is assigned to a single element of 13. In the function: Let f be a function from A to B, then the function f is said to be a function if every element of B is assigned. Set with total order: If P is a set in which every two members are comparable, it is called a set of complete order or toset or a chain.
Maximum element: An element a of a set P is called a maximal element of P if a Meet semi Lattice: A non-empty set P together with a binary operation A (measure) is called a meet semi Lattice if for all a,b,c E P,. Part semi-lattice: a non-empty set P together with a binary operation v (merging) is called a measure semi-lattice. Convex sublattice: A subset K of a lattice L is called a convex sublattice if for all a,b c= K,[aAb,avb]cK. A lattice L is called a complete lattice if for every subset K both sup K and inf K exist in L. Filter or double ideal: A non-empty subset I of a lattice L is called a double ideal of L if a, b E I implies that a A b E I. In every element x in an interval [a, b j has at least one complement with respect to [a, b], the interval [a, b] is called complemented. Furthermore, if every interval in the network is complemented, the network is said to be relatively complemented. Based on this finding, the concept of a network with sectional antitone involutions was defined in [4] and [5]. Introduction: In this chapter we study antitonic and sectionally residual networks. First, lattices with the largest element I where in each interval [a, 1] an antitone bijection is defined. We characterize these lattices by means of two induced binary operations that prove that the resulting algebras form a manifold. These meshes also have the property that an antitonic involution is defined in each of their main filters. In this paper we present a further generalization of this concept, defining the notion of a lattice with antitonic sectional bijections. In this short note we will compare a certain modification of a residual network with the concepts already introduced (see [2, 8]). Therefore, x=loz:!~xoz, forever x,zEA and so. so f0 and f are bijections in [a,l]. and reverse each other). It is very easy to see that in the presence of distributivity (a, b) is an ideal of L. Ir We also include characterizations of modular and distributive lattices in terms of relative annihilators. In section two, we have introduced the concept of relative stone lattice and generalize several results of. Double atom: An element b is called a double atom if u, the largest lattice element covers b. L is finite and sectionally complete => every nonzero element C of L is a union of many finite atoms. Theorem: 3.4.2 (representation theorem) Let B be a finite Boolean algebra, and let A denote the set of all atoms in B. We know that every v e B can be expressed as a union of many atoms: v = a1 v..v a with all atoms a. Introduction: In lattice theory there are different classes of lattices known as manifold lattices. A bounded distribution lattice L is called a pseudo-complemented distribution lattice if every element of it is pseudo-complemented. As a partition grid, it has twenty-five subgrids and eight congruences; if a lattice with pscomplementation has three sub-algebras and five congruences. Proposition 4.2.2 Show that in a stone algebra every prime ideal contains exactly one minimal prime ideal. Proof: Let P be a prime ideal and q1 and q2 be two minimal prime ideals containing i p with q1 * q2,. Normal lattice: A distributive lattice L with 0 is called a normal lattice if every prime ideal of L contains a unique minimal prime ideal. Proof: Since LF is a distributive lattice. yAfAgyAzAfAg, and yAfAg=yAZAfAg, andso xAfAg:!~yAfAg:5zAfAg, that is, xAh:!~yAh:5zAg where f A g E F. Assume that £ is the relative pseudocomplement of y A h i [x A h, Z A h ]. Introduction: it is shown that any direct mapping equipped with intersecting switching maps can be represented as a certain implication algebra. QuackenbuSh [191 due to the axiomatization of algebraic structures defined by an upward ordered set. B;::!~,l) will be called by sectional switching involutions if there is a sectional switching involution on the section [p,1] for every p E B . Let us note the name implication algebra expresses the fact that x a y is interpreted as a binding implication x y. Then D(B) = (B;v) is a directoid of largest element 1 with section-changing involutons whose induced order coincides with that of B. Since the section map on [y,l] is an involution, we derive xoy=(xoy), =(xoy)oy)oy=(xvy)o=yoy=l. Let :5 be its induced order and for each p e C there exists an involution of the sectional permutation map x i—* x" in [y,l]. We will obtain the conditions for the antitone of the permutation map and it appears a link with completion in sections. We study the following notation: for each a e L and x € [a,!] we denote by x° the image of x in this cross-sectional mapping onto [a,l]. So if the sectional mapping is an involution, we conclude, (xvy)vy=((xvy)VYY =(xvy) =xvy,. ii) Let every sectional mapping be weakly switching, let 1 E L and x E Then xvl=x author( i). Proof: Since antitone involutions switch maps in sections, by Lemma 6.1 .1 and Theorem, x' is a lattice and is a complement of.Algebraic Lattice
Lattices with sectionally antitone bijections
Sectionally residuated Lattice At first, we recall the basic concept
It 3.1 Some characterizations of relative annihilators in Lattice
Relatively complemented Lattices
Pseudocomplemented Lattice
Basic concepts
Basic concepts
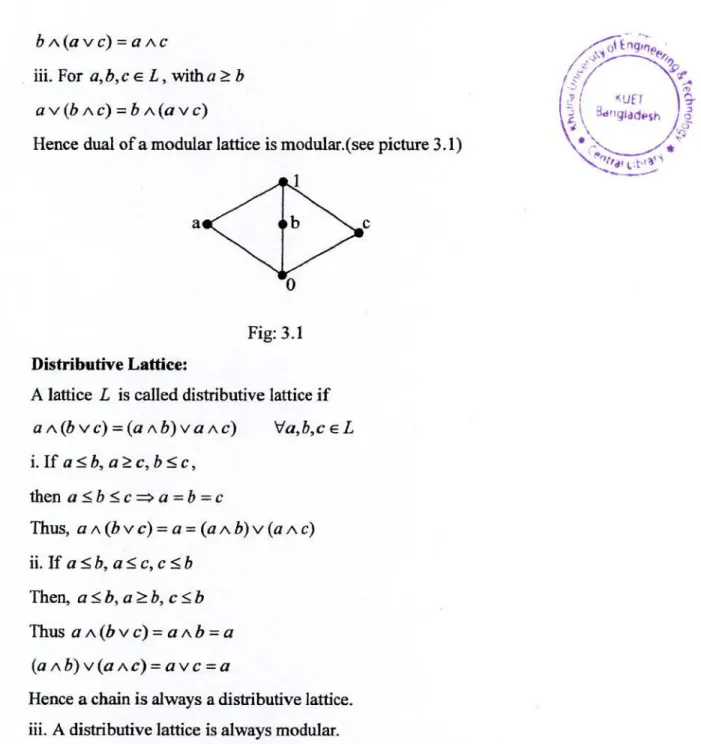
The compatibility condition