Faculty of Cognitive Sciences and Human Development

Faculty of Cognitive Sciences and Human Development. INVESTIGATING STUDENTS' UNDERSTANDING OF LINEAR. EQUATIONS BASED ON SCHOENFELD'S MODEL. Nurulaheila Billti Jalaludin. Bachelor of Education Mathematics with Honours QA 11.2 N974. 2008. 2008.

l'u 1'". INVESTIGA TlNG STUDENTS' UNDERSTANDING OF LINEAR EQUATIONS BASED ON SCHOENFELD'S MODEL. NURULSHEILA BINTI JALALUDIN. Thi s projec t is submitted in partial fulfillment of th e requirements for a. Bachelor of Educatio ns with Ho no urs. (Mathematics). Fac ult y o f Cognitive Science and Human Development. UNlMA S.

The project entit led ' Investigating Students ' Unders tanding of Lin ear Equ atio ns Based on Schoenfe ld 's Mo del' was prepared by Nuruls he ila binti .Ialaludin and submitted to the Facult y of Cognitive Science and Human Deve lop me nt in partial fulfillm ent of the requirements for a Bac helor o f Educa tio ns (Mathematics) w ith Ho no urs.. Rec eived for examrnatio n b. (Mr. Philip Nuli Anding) Date:. ____ ~~h_~_h t ______ _. G rade. A­.

BORANG PENGESAHAN STATUS TESIS. Gred:. A~. nmUL INVESTIGATING STUDENTS' UNDERSfANDlNG BASED ON SCHOENFEL D'S MODEL. SESI PENGAJIAN 2007/2008 Saya NURU LS HEILA JALALUDIN mengaku membenarkan teSIS * ini disimpan di Pusat KJ1idmat Maklumat Akademlk, Universiti Malaysia Sarawak dengan syarat-syarat kegunaan sepertl berikut: I. Tesis adalah hakmilik Universltl MaJays13 Sarawak 2. Pusat Khidmat Maklwnat MadernIk, Universlti Malaysia Sarawak dibenarkan membuat salinan untuk tujuan pengajtan sahaja 3. Pusat Khidmat Maklwnat Madernik, Umversitl Malaysia Sarawak dibenarkan membuat pendigitan untuk membangunkan Pangkalan Data KandWlgan Tempatan 4. Pusat Kludmat Maklwl1at Akademik, Universiti Malaysia Sarawak dlbenarkan membuat salman teSlS ini sebagai bahan pertukaran antara U1StltUSI pengajian tlUggl 5. *' sila tandakan ( ,f). [J. SULIT. [J TEREAD. (MengandWlgl maklumat yang berdarjah keselamatan atau kepentingan seperti telmaktub di dalam AKTA RAHSIA RASMI 1972) (Mengandungi maklwnat Terhad yang telah dltentukan. oleh organisasi/badan dl mana penyelldikan diJ31ankan). TIDAK TEREAD. (TANDA ANGAN PENULIS) . (TANDATANGAN PENYE LlA). No 75. Jalan Ambar 5, Taman Ambar, 43800 DengkiI, Selangor.. Tarikh.. ''lankh. Catatan:. * Tesis dlmaksudkan **. ~f (jo f •. sebagai tesis bagi Ijazah Doktor Falsafah, Sarjana dan Sarjana Muda Jika tesis ini SULIT atau TERHAD, sila Jamplrkan surat daripada pihak berkuasa/organisasi berkenaan dengan menyatakan sekali sebab dan tempoh tesis iIll perlu dlkelaskan sebagal TEREAD..

ACKNOWLEDGEMENT. I wo uld like to dedicate this acknowledgeme nt to those w ho s upponed me directly and indirectly in preparing thi s fmal year project. first o f a ll, I would like to thank A llah S WT for His Grace and Blessing in g iving me strength and spirit in comple ting thi s researc h. Spec ial thanks al so go to my parents, hro ther, sister and o ther famil y members w ho suppolted and enco uraged me. I wo uld like to express m y spec ial thank s and gratitude to the Principal of Seko lah Menengah Sain s Kuching for givin g me the opponu nit y and allowing me to co ndu ct th is stud y and not forgetting th e teachers and also stud cnt s of Sekolah Menenga h Sains Kuching who are the respond ent s of thi s stu dy for their cooperation and the ir time in completing the questions and int erview . I sincerely appreciate their commitment in helping me complete this study. My apprec iation also goes to my superviso r who helped me a lot through his guid ance and co mments in the preparation and co mpletion of this proj ect. Thank you for the commitment and contribution. Last but not least, special thanks a lso go to my friends and my course­ mates fo r the ir enco uragement, advice and suppo n pen ain ing to m y research.. IV.

l'u'a!. KllIOmal \JakluII'31. ll~l\El{$lTllAl.AYSl,\. "'''''aenu". SARAWA¥. \.I"':' ~tl"'_ ~(lfJ ~ !nl~r'ltI"". TABLE OF CONTENTS j ii. iv. Acknowledgemen t Table of Contents List of Figures Abstract A bstrak. vi. vi i. viii. CHAPTER 1: INTRODUCTION. 1.1 1.2 1.3. 14. 1.5 1. 6 1.7 18 19 1.10. Introduction Backgro und of study Theories ofhuman information processtng 1.3.1 Schoenfeld's Model of Mathematical Co gnition 1.3.2 Atkmson and Shiffrin: Multi sto re Mode l 0 f Memo ry Prob lem Statement Objectives of Stud y 1.5. I General Objectives 1 5.2 Specific Objectives Resea rc h Question Defmiti on of Terms 1.7.1 Conceptual Definitio ns 1.7.2 Operational Definitio ns Co nceptual Framework Sig nificance of the Stud y Limitatio n of Stud y. I. 3. 5. 5. 9. 10. 12. 12. 12. 12. 13. 13. 15. 16. 17. 18. CHAPTE R 2: LITERATURE VIEW. 2. J 2.2. 2.3. 24 2.5 2.6 2.7. Introduction Students Knowledge 0 f Mathematics Thinktng Skills in Mathematics Knowledge and Understandmg of Lmear Equation Scho enfeld 's Model of Mathematical Cognition Problem-Solving Strategies The o ther Related Research. 19. 20. 21. 22. 24. 25. 26. CHAPTER 3: METHODOLOGY. 3.1. 3.2 3.3 3.4 3.5. 28. 29. 29. 31. 32. Introd uction Location o f Study Populatio n and Sampling Operatio nal Framework Resea rc h Instr ume nt. v.

3.5. 3.6. Res earc h Procedures 3.5.1 Think Aloud Me th od 3.5.2 Written Answer 3.5. 3 Interview Data A nalysis. 33 33 35. 35 35. CHAPTER 4: RESULTS AND FINDINGS 4. 1 4.2. 4.3 4.4. In tro ductio n Res ults 0 f Analysis 4.2. 1 Conceptual hllO w ledge ofLi.n ear Equation 4.2.2 Others Concep tual hllOwledge 4.2.3 Procedural Knowledge in L inear Equatio n 4.2.4 Others Procedural Know ledge Problem-so lv i.ng St rategies St udent 's Model o f Understanding 4.4 . 1 Model I 4.4.2 Mode l 2 4.4.3 Mode l 3 4.4.4 Mode l 4 4.4.5 ModelS 4.4.6 Mode l 6. 37 38 38. 40 43. 48 51 51 52. 53 54 56 58. 69. CHAPTER 5 : DISC USSION AND CONCL US ION 5. 1 5.2. 5.3. 5.4. Introduction Discuss ion Recommend ations 5.3. 1 Teacher and Teachi.ng Process 5.3 .2 Students and Learning Pro cess 5.3.3 Others Researchers Conclusio n. 61 62 64 64 64 65. 66. Refe rences. 67. App e ndix. 71. Vi.

LIST OF FIG URES. Figure 1.1 Atkinson and S hi fTrin's Model. 10. Figure 1.2 Conceptual Framewo rks. 16. Figure 3.1 Operational Frameworks. 31. Figure 4. I Modell. 52. Figure 4.2 Model 2. 53. Figure 4.3 Mode l 3. 55. Figure 4.4 Model 4. 57. F igure 4.5 Mode l 5. 58. Fig ure 4.6 Model 6. 60. VB.

ABSTRACT. INVESTIGATING STUDENTS' UNDERSTANDING OF LINEA R. EQUAnONS BASED ON SCHOENFELD'S MODEL. Nurulsheila binti Jalaludin. This researc h is ca n'ied out to in vestigate Form T wo students' understanding of Linear Equations based on Schoenfeld's model. Schoen fe ld 's model is a model about mathematics thinking which includes four informatio n proc ess ing components which are (1) the knowl edge base, (2) problem-solving strat egies, (3) mo nitoring and control and (4) beliefs and affect s. This research applied only th e first two components of Schoenfeld 's model. This research investigates wh at are the conceptual and procedural know led ge used by students when th ey an swer questions about Linear equations, stude nt s' pro bl em-sol ving strategies and develo p models of students' unde rstanding of Linear Equations. Data co llectio n in vo lves the metho d of triang ulatio n w here by three types of data coll ected a re the think alo ud data, wlitten docume nt and interview. S ix questions from the to pic o f Linear Equations are cho sen and used to investigat e Fo rm T wo student s' unde rstanding o f Linear Equations. The analys is o f data indicated that there are four conceptual a nd six procedural knowledge used by stud ents in o rd er to answer questions abo ut Linear Equat ions. Conceptu al and pro cedlu'al know ledge o f fractions and perimeter are also used by students. The finding s generate s ix models of Form Two students' understanding of Linear Equations. This researc h is relevant to both teachers and students in teaching and learning o f Linear Equ ations.. VlIl.

ABSTRAK. MENGENALPASTI PEMAHAMAN PELAJAR DALAM TOPlK PERSAMAAN. LINEAR BERDASARKAN MODEL PEMIKlRAN SCHOENFELD. Nllrllisheila binti Jalalud!". Kajian ini dijalankan unlllk mengenaipasli pemahaman pelajar-pe/ajar lingkalCln dua dalam topik Pelsamaan Lineal' berdasadan model pemikimn Schoenfeld. Model pemikiran Schoenfeld lerdiri dar/pada empa l kompol1 en p emprosesan moklumCil iailll (!) asas pengelClhllan termQSlIk pengelahllal1 konsep dal1 pengelahual1 prosedur, (2) lal1gkah-lal1gkah pel1yelesaian masaiah, (3) pemerhalial1 dan p engawasan dan (4) kepercoyaan dan kesan-kesannya. Kajian hanya mengambilkira dua komponen 1.ltama dalam model pemikiran Schoenfelc/. Kajian ini cuba mengenalpasli pengelahuan konsep dan prosedur yang digunakan oleh para pelajar unlllk menjawab soalan berkenaan Persamaan Lineal', langkah -Iangkah penyelesaian masalah yang digl.lnakan yang mana pengkaji menggLillakan slralegi Polya sebagai panduan dan membenLUk model pemik iran p elajar. Dalam proses pengumpulan data, pengkaji mengaplikasikan kaeda h 'triangulatian ' yang terdiri daripada kaedah 'think alolld', dokl1lnen berllliis dan temllbLial. Ena", soala" dari iOpik Persamaan Linear ini dipilih berdasarkan sLikalan pelajoran Malema tik tingkalan dlla. Rerdasarkan dapalan kajia n, terdapal empat pengelahuan konsep dan enam pengetahua n prosedllr yang digunakan oleh pelajar-pe lajar unlllk menjawab soa lan herkenaan Persamaan Lineal'. Para pelajar juga mel1ggllnakan pengela huan kon sep dan prosedur lambah an seperli perimeler dan pecahan. Hasil/rajian membolehkan pengkaji menghasilkan enam model pemikiran pelajar berkenaan lopik Persa maan Linear yang melibalkan prosedur yang belli I dan allemalif prosedur. Dopatan kajian ini jelas secorCI konseplual dan relevan kepada gum-gu ru dan para pelajar dalam proses pengajaran dan pembelajaran.. IX.

CHAPTER 1. INTRODUCTION. 1.11ntroduction. Education is very impor1ant as it serves to complement national socio­ economic development. Science and technology are crucial aspect to be learnt by the students including mathematics because it is a par1 of science. According to Curriculum Development Center that is CDC (2004), mathematics which is taught in primary and secondary schools are very important as it gives opportunities for the students to develop knowledge and skills of mathematics as well as promote higher order thinking skills, problem solving skills and applying mathematics in daily lives..

Learning mathe matics is not just accepting the knowledge 6'o m the teac hers, It. requires stud ent s to explore, think, discover, adapt, modify and be inno vative to. change and face future c hallenges.. Research done by Tella (2007) in contemporary Nigeri a stated that development of Industrial and Technological fi e ld caused students to take up sc ience and related subjects espec ially mathe matics. Mat hema tics is ver y important beca use it co ntributes to ward s eco no mics deve lopme nt o f a co untry. Therefo re, mathe matics is compu lsory at all levels of schooling. Unfortunately, the result reported that students' perfo nnance in mathematics at the end of seco ndary school has not improved in the past decade (Tella, 2007).. In Malays ia, mathe matics' curriculum faced so man y c hanges up to thesc da ys . The develop ment of mathematics cu nic ulu m is ca used by the deve lopment of new concepts and techniques that changed the mathematics structure in late nineteenth century (Khoo, 1986). Furthermore, spec ial development of mathematics' curric ulum had a big impact on the success fuln ess in many areas which involved mathematics such as biology, social science and eco nomy. Othe r than that , deve lopment and extend ed use o f math ematics impl y an inc rease in th e needs of spec ialist in sc ience and mathematics (Khoo , 1986).. In mathematics, th ere are many topic s to be learnt and taught at different ages. In U nited States, the sub-topics of aritlunetic's and algebra llke addition and s ubtraction are taught at the early ages that are 5 to 7 years o ld . At the age o f 7 to 8 yea rs old , childre n start to learn multipl icat io n and div ision (Howe, 199 1). This co ntinues with pre-algebra and Algebra I at th e age of II to 13. Geometry would be the next topic at th e age of 14 and continu es by Algebra II at the age of 15. The harder topics such as trigonometry and calculu s are the last, for students aged 16 and 17 (Howe, 1991 ). These levels were organized acco rdingly to stude nt s' leve l of.

acceptance o r se lf-development (Wikipedia, 2007c). As the chi ld ren get older, they are increasingly likely to make use of strategies and learning plans for effectiveness of learn ing other topics (Howe, 1991).. In Malaysia, knowledge of mathematic s start at an ea rl y age of 6 ycars old at pre-school, then up to primary school, secondary sc ho ol a nd university levels. Learning at seco ndary school leve l is divided into two which are lower and upper form s. Thi s research focuses on the lower fOlms and concerns the topic of Linear Equ atio ns. The topic of Linear Equatio ns topic is after the top ic of A lgebraic Exp ressio ns J a nd the fourth topic in the math e mat ics cUITicul ar for Form T wo stud ent s.. Linear Equations require so me. basic. know ledg e of algebra. and. mathe mat ica l operations such as addition, subtract io n, multiplica tion and divis ion. Important parts that should be learnt fU'st are the ternlS used in this top ic and the meaning of symbo ls such as equal sign, plus, minus , divisio n and multiplication signs. At this level, only three learning objectives are stated to be accomplished. The first is to understand and use the concept of equa lity. Second, is to und ers tand and use the concept of lin ear equations for one unknown variab le and la stly is to und erstand th e co nc ept of so lutions of linear equations for one unknown variabk (Curriculum Development Centre, 2004).. There are many ways oftcaching and leaming mathema tics (Woo lfo lk , 2004). The effectiveness o f one style depends on the abilit y of students to accept it. Acco rding to Hiebert (1992), learning mathemati cs had a commo n accepted goal amo ng mathematics educators that student s sho uld understa nd mathe mat ics and being apa rt of the learning process. Constructivi sm is the most co mmonly used by mathemat ics ed ucators whe reby students participate act ively in the classroom setting so that they ca n develop the ir own understandin g o n mathemat ics (Van de Walle, 2004).. 2.

1.2 Background of Study. T he trad itio nal method of teaching in the c lassroom does not prepare stud ents to the modem world . Teacher should g ive mo re spaces for students to develop the ir own und erstandin g a nd ha ving stud ent-cent ered classroom is a better way of teachin g nowad ays. Indi vidua l characteristics suc h as inte ll igence, cognitive styles and person ality play important roles in leaming and influence academic achievement (Tella ,2007).. Piaget in his work in 1969 emphasizes on how c hildren go about making se nse o f their wo rld by gathering and orga niz ing information (Woo lfolk, 2004). According to Piaget, there are four stages o f cogniti ve develo pment whic h a re se nso rimotor fo r the age gro up o f 0 to 2 years o ld, preoperatio nal stage for ages 2 to 7 years o ld , co nc rete o perational stage for ages 7 to II years o ld and fOlmal operationa l stage fo r I I years old to adults. At operational stage , children are able to assimilate new in formation into existing schemes and accommodate children altering exist ing schemes or create new ones in response to new info nnation (Woolfolk, 2004). These abiliti es help stud ents learn mathematics since lea rning mathematics is no t just accepting information but depends on learner's activ ities and eftorts.. Mat hematics lea rning no wadays seems more o n critica l thinkin g w here by students develo p their own und erstanding o f mathe matics (Tella, 2007). Developing our own understanding or kno wledge is the basic idea of co nstructivism. Cognitive constructi vism,. ground ed primarily in Piaget's. work,. emphasizes. individu al. constru ctio n of understand ing in a situation. At this stage, soc ial interactions amo ng student s offer opp ortunities for them to gain new know ledge and understanding of new situation (Piagel, 1976) . Social constructi vism, based o n the Vygotsky's work suggests that th e process of soc ial interac tio n is important in learning because kno w ledge is influ e nced by a n envirorunent, that is initia ll y ex ists in the social. 3.

environment , and it is even tually internalized by individuals (Vygotsky, 1997) . For exa mple, student s try to co nstruct their own und e rstanding of linear equatio ns based on the kno w ledge that they ha ve in the prev io us lesson w hich arc a lgebra ic equations in linear fOlm and other mathematical operat ions.. The knowl edge that the student s received in the classroom ent ers the process of information processing w here it tend s to explain how human acquire information, son and organize info rmatio n or kno wledge, and how to retrieve the knowledge w hen needed. There are three ass umptions propo sed on behavioral basis of information­ processing processes. T he fu'st assumption is that information is processed in stages;. (1) attending to sensory input, (2) transforming the input to mental image, (3) co mparing ment a l image with information a lready s tored in memory, (4) assigning meaning to image and (5) acting on the image in some way. The seco nd assumptio n states that there arc limit s at every stage w he re the information ca n be processed e ven though there is an inftnite amount of knowl edge that human can attain at one time. The third assumpti on is about the idea of human information-process ing system which is consid ered as interactive. The learning could occur when there is an int eraction between an env ironment input and lea m ers who process or trans form the information (Hamac hek, 1994 ; Miller, 1983).. Information-processing. helps. us. remember. This could be c learly defIned. und erstand 111. how. students. learn. and. SO me models of human infolmation. process ing like Schoenfeld' s model or Atki nso n and Shiffrin's model that wou ld be discussed next.. 4.

1.3 Theories of Human Information Processing. There are several mod els that can be used to in vestigate students' understanding of Linear Equations. In this research, Schoenfeld's mod el of mathematical cognition is taken as the main model. Other related theories that would suppOJ1 this study are Atkinson and Shiffrin multi store model of memory and problem so lving.. 1.3,\ Schoenfeld's Model of Mathematical Cognition. Schoenfe ld 's model is one model abo ut thinking. Schoenfeld proposed his model of thinking a few decades ago. Sc hoen fe ld 's model includes four infonnatio n processing components which are (I) the kn ow ledge base, (2) problem-solving strategies, (3) monitoring and control and (4) beliefs and affects (Pressley & McCormick , 1995). T here is also a fifth component which relates to instructiona l practices that promote effective mathematical cognition. Schoenfeld's model also involves the information processing hardware which are short-telID and lo ng-t erm memories. Natio nal Co uncil of Teachers. 0. f Mathe matic s o r NCTM Standards ( 199 1). co nsidered all o f the fa c tors which Sc hoenfeld stated as being impol1a nt in mathematics thinking and problem-so lvin g. [n 1987, Sc hoenfeld came out with a literature that shows the task of metacognitive refl ec ting upon students' existing conceptions and can fo ster students' critical, analytical , and reflective thinking. Students can evaluate these levels of und erstanding by discussing; interacting and negotiating meaning s through appropriat e learning activities on student s cent ered formed (Afamasaga-Fuata'l, 2006).. Sch oenfeld 's model o f mathematica l cognitio n co uld be co nc lud ed as co nsistent and a good information process in g model s ince it lnvolves know ledge base, pro ble m- so lving strat egies, monito ring and control and beliefs and affects. It. 5.

has a big impact on the development of new models of problem-solving instruction (Pressley & McCormick, 1995), In this study, researcher focuses only on the two components of Schoenfelds' model. 0. f mathematical cognition.. (a) The knowledge base. Knowledge in Schoenfeld's model involved both declarative and proccdural knowledge, Declarative knowledge deals with factual information that involves knowing "what" while procedural knowledge deals with the knowledge of how to do things which usually concerned solving problems (Pressley & McCormick, 1995). ) There are many differences. between procedural knowledge and. declarative. knowledge. Basically, declarative knowledge could be proved by variety forms such as recall, recognition, application, association to other knowledge and it acquired exposurc. in. leaming.. On the other hand,. procedural knowledge could. be. demonstrated by only performing the procedure and it has no right or wrong answer as far as the value of procedural knowledge is determined aher extensive practice (Pressley & McCormick, 1995; Schoenfeld, 1989).. Both declarative and procedural knowledge are stored in long tern1 memory which has unlimited spaces for storing information, When students deal with the knowledge that seems familiar with what they had already known, procedures operate on declarative information in working memory or shol1-term memory. Shon-term memory is characterized as limited capacity system and handles information that is to be stored for a shon period (Houston, 1991). But if the knowledge is ncw to the students, it will try to fit with the existing schemes and stored in long-term memory (Houston, 1991).. 6.

(b) Problem-solving strategies. Prob lem ca n be defined as "something that exists when a motiva ted organism. is trying. 10. reach a goaL but is blocked /1-0111 doing so by an obswcLe or obstacles ". (Houston, 1991), A problem may be simple but some might be very co mpl ex , To so lve problems, there are many ways that could be used whether heuri stics problem so lving or algorithmic pro blem so lving (Houston, 199 I), Basica ll y strategies for problem so lving included understanding the prob lem, so lving the probl em and re fl ec ting on the answer and solution (Press ley & McCo rmick, 1995),. In Schoenfeld 's model of cognition, problem- so lving strategie s that used are Polya 's strat egies, Po lya 's s trategies co nsist o f four steps w hic h are; (i) ana lyzing th e problem, (i i) c le arly stat ed goals or needs o f the problem, (iii) plan a nd carr y o ut strategies and (iv) loo k back strateg ies o r veri fY ing the so lutio n (Pressley & McCormick, 1995),. Analyzing and understanding the probl em are the most imp 0l1ant aspects of problem so lv ing especia lly in mathematics because it is actually about explaining the question 's need, Stated goal, plan and carry out strat egies are the next phase includi ng a discussion. 0. f methods used, Mathematics problem usu all y need students. to draw a diagram based on the information given, then loo k for patterns su ch as pat terns of numb er and operations, Some students may need to mak e a [abl e o r chart to combinc with pattern searching as a means of solving problems or constructing new ideas, Ot her than that, there is also the st rategy of making guesses and c hecks tllrough tri al and error. Lastly, students may use an organized list or teacher's notes as references, Looking back strategies s hould be done atier a solution has been fo und in order that stud ents are able to know their mistak es and ab le to improve the ir skill s in mathematics (Va n de Walle, 2004). 7.

(c) Monitoring and control. Solving problems also relates to metacognition which refers to conscious monitOling and regulation of one's own thought. According to Schoenfeld (1989), a good problem solver monitors their thinking regularly and automatically; "They make. conscious decisions to switch strategies, rethink the problem, and search for related content know/edge that may help, ur simply start af/"esh". Schoenfeld highlighted monitoring as a contribution to self-regulated use of knowledge, including procedural knowledge. Teachers play major roles to coach students during their problem-solving attempts; pose questions in order to sensitize students and become aware of the problem solving strategies and the effects of the strategies they are using (Van de Walle, 2004).. In becoming aware of the strategies used, students acquire metamemory skills that allow them to be good at knowing when the appropriate time to use strategy, choosing appropriate strategy and applying the familiar strategies to unfamiliar situations or problems (Howe, 1991). In older children and students, the learning capacities and experiences become wider and better at monitoring and control of one's own thought (Howe, 1991). Schoenfeld believes that teachers can improve students' understanding of mathematics and self-regulated problem solving by given some space for the students to monitor the mathematical concepts and strategies as well as coaching students to monitor and self-regulate even though it takes a long period of time (Pressley & McCormick, 1995).. (d) Beliefs and affects. Students usually construct. beliefs about. mathematics. based on their. experiences in the classroom These beliefs concerned on their abilities to do mathematics and to understand the nature of mathematics tbat have a significant. 8.

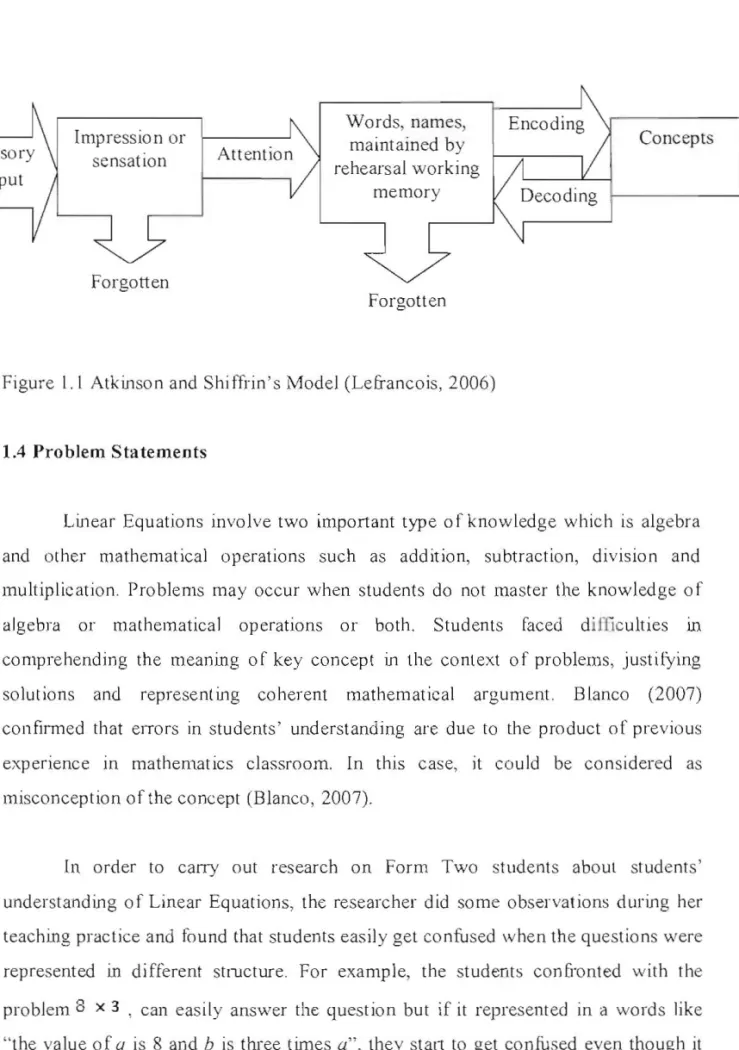
effect on how students approach problems and how well they succeed (Van de Walle, 2004). For example, some students might conclude that learning mathematics in school is more about memorizing than reflecting and therefore, the best way to do well in math is to memorize all the formulas. This kind of beliefs has higher potential for discouraging reflective problem solving and students are more likely to follow all thc rules stated by the teacher and became discourage to think by themselves (Pressley & McCormick, 1995).. Beliefs affect motivation and hence determine behavior about mathematics. Students who think that mathematics was just memorizing formulas will become permanently unmotivated to think when encounter with problem solving situation. But students with strong beliefs that mathematics problems can have many alternative ways of getting the one and only right answer will become motivated and reflective problem solver who persist in the face of difficulties during problem solving (Pressley & McCormick, 1995).. 1.3.2 Atkinson & Shiffrin: Multi store Model of Memory. Another model which relates to information processing is Atkinson and Shiffrin's model, also known as modol model. This model focuses on the differences between short-term and long-term memory as well as the third component relating to sensation callcd sensory memory (Lefrancois, 2006).. In the Atkinson and Shiffrin's model, sensory information first enters at sensory memory. Then the information gets into working memory. also known as short-term memory where it is available as a name, a word or an image. Not all of the information in short-term memory could pass through long-term memory, only some may be encoded for long-term storage, where it might be available for retrieval into sh0l1-term memory when needed (Lefi'ancois, 2006).. 9.

Senso ry Input. Words, names, maintained by re hearsa l working me mory. Impress ion or se nsation. Concepts. Forgotten Forgotten. Figure I. I Atkinson and Shi ff!'in's Model (Lefranc ois, 2006). 1.4 Problem Statements. Lin ear Equations involve two important type of knowledge which is algebra and ot her mathematical operations such as addition, subtraction, division and multiplication. Problems may occur when students do not ma ster the knowledge of algebra. or mathematical. operations or both.. Students. faced. difliculties. in. co mpre hending the meaning of key concept in the context of problems, justifying so lutions and. representing. coherent. mathematical. argument.. B lanco. (2007). con finned that eITors in students' understanding are due to the product of previous expenence m mathematics classroom. In this case, it could be considered as misconception of the concept (Blanco, 2007).. In order to cany out research on Form Two students about students' understanding of Linear Equations, the researcher did some observations during her teaching practice and found that students easily get confused when the questions were represented in diffe rent stmc ture. For example, the stud ents conlionted with the problem 8 x 3 , can eas ily answer the questio n but if it represented in a wo rd s like "the va lu e of a is 8 and b is three times a", they stan to get co nfu sed even though it obv io usly relates to the same answer (A llsopp, 1997). This is actua lly related to the. 10.

problem of memory where students have faulty or inefficient memory retrieval mechanism. Furthermore, students faced problem when they are confront ed with multi- step problem so lv ing situations whereby students cannot relrieved from memory what they should do next (Allsopp , 2007).. Other Ihan Ihal , students find it difficult to explain and justify their a nswers mat hemat ically in ter ms of the conceptual structw-e ofa topic . This us ua ll y happened when the questions need to be explained and so lved qualitatively. Thi s ma ni festatio n is typically a co mmunication problem resulting from student s' inability to und erstand the meaning of a language that is concepts, principles, theo rems and theories used in mathematical discussions and dialogues (Afamasaga-Fuata'l , 2006)_. Schoe lfeld (1994) added that students tend to use any procedure to gel an answer without really checking whether an algorithm is suitable to the problem. On the other hand, Schoelfeld reported that professional spends more time analyzing problem before making the fu-st move to solve a problem (Pres sley & McConnick , 1995; Schoelfeld, 1994)_. Lastly, beliefs of mathematics differ between students. Beliefs are primarily about ''"vhat is tru e?" and when it is related to mathematics knowledge, it could be such "Is it possible to solve mathematics. problel1~5. with more thon olle method)". (LovelL 2002)_ Beliefs affect motivation and this will result behav ior. I f the students are taught us ing traditional method , the learning pro cess mi g ht not he lp in improving student s' way of thinking _Students should be given oppoI1unities to work beyo nd the textbook and exam ination style of questions as to look forward a nd can see their stud y in cont ext (Lovell, 2002).. II.

1.5 Objectives of Study. 1.5.1 General Objectives. The main purpose of this study is to investi gate student s' understanding of the to pic o f Linea r Equations based on the two steps in Sc hoenfeld's model. The two steps in Schoenfeld' s model include the knowl edge based and problem-solving strategies. The prob lem-solving strategies are also referred to as Po lya 's strategies.. 1.5.2 Specific Ohjectives. In this stud y, the spec ific o bjecti ves are : 1. To ide nti fy the co nceptual kno w ledge that students used whe n they answer the qu estions about Linear Equatio ns 2. To id enti fy the procedural knowledge that stud ent s used when they answer the qu estions about Linear Equations 3. To id entify the strategies that students used to answer qu estions about Linear Equ atio ns 4. To deve lop model of students' understanding o f L inea r Equ atio ns. 1.6 Resea reh Questions. In co nduc ting this investigation, there are fo ur questio ns posed as guidance ttu-o ugho ut th is stud y. These are as follo ws : I. What is the co nceptual knowledge stud ents used in order to answer questions about Linear Equations? 2. Wh at is the procedural knowledge stud ent s used in ord er to answer questions abo ut Linear Equations?. 12.

3. 'Nhat are the strategies students used when answenng questions in Linear Equatio ns? 4 . What kind of model could be constructed to rep rese nt s tud ents' knowl edge of Linear Equations'). 1..7 Definition of Terms. 1.7 ,1 C onceptual Definitions. (a) Linear Equations with One Unknown. According to Wikipedia (2007 b), a linea r equatio n is an equ atio n in w hich each term is e ithe r a co nstant or the product o f co ns tant times the fIrs t power of a variable. T hese equatio ns are ca lled "linea r" beca use they represent stra ig ht lines in Catiesian coordinates. Linear Equations w ith one unknown are the equ atio n co nt ains an aritrun etic operation with one variable and numbers (Bo go molny, 2008). It only has one so lutio n and to lind the solution students need to " undo " whatever has been done to the vari ab le. Students have to remember that whatever th ey do to the one sid e, they must do the exac t sa me thing to the other side of an equatio n and this is the concep t o f equality (S tap le, 2007).. (b) Conceptual Knowledge. Co nceptu al knowledge generally called declarati ve know ledge is the knowledge of fact s, the mea nings o f symbo ls and the concepts and principles o f a particular fi e ld of stud y ( Hiebert & Lefevre, 1986). Conceptual kno wledge is a k now ledge that is ric h in relatio nships. It can be tho ug ht o f as a co nnected we b of kno w ledge, a networ k in whic h the linking re lat io ns hip s at'e as promine nt as the discrete pieces o finfo rrnation .. 13.