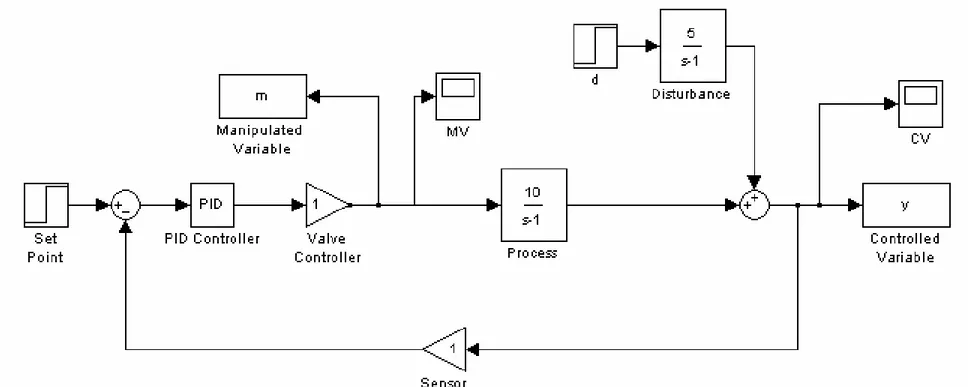
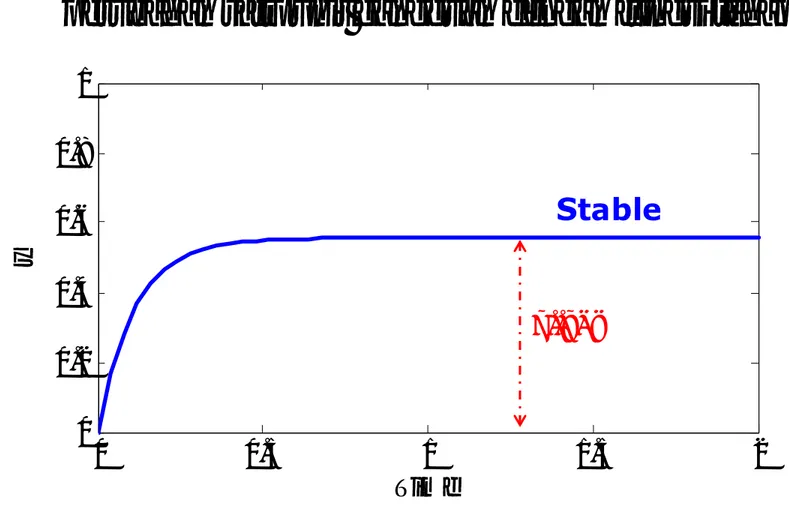
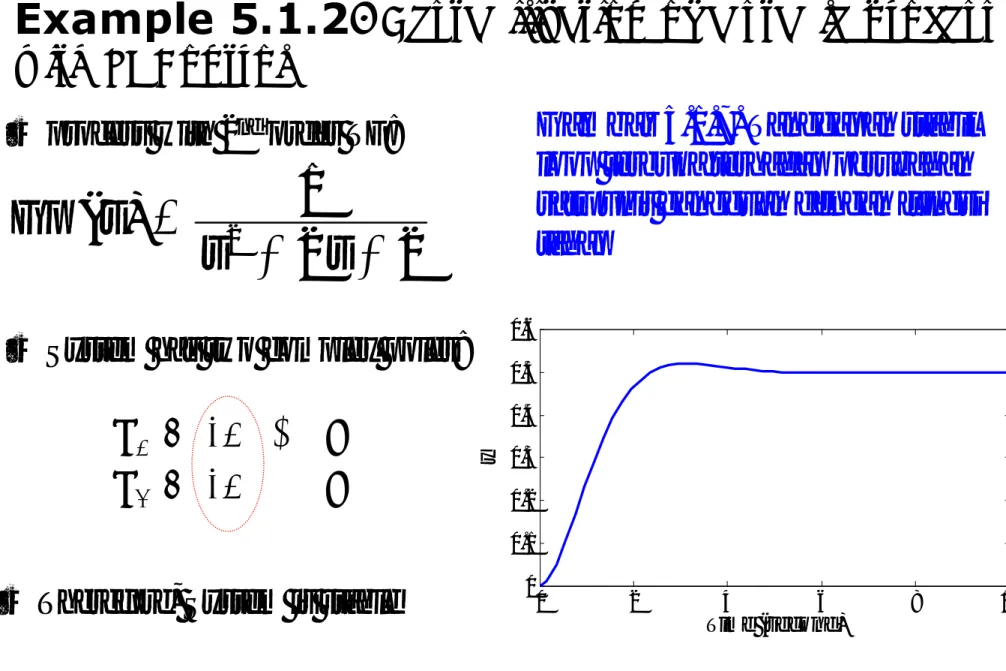
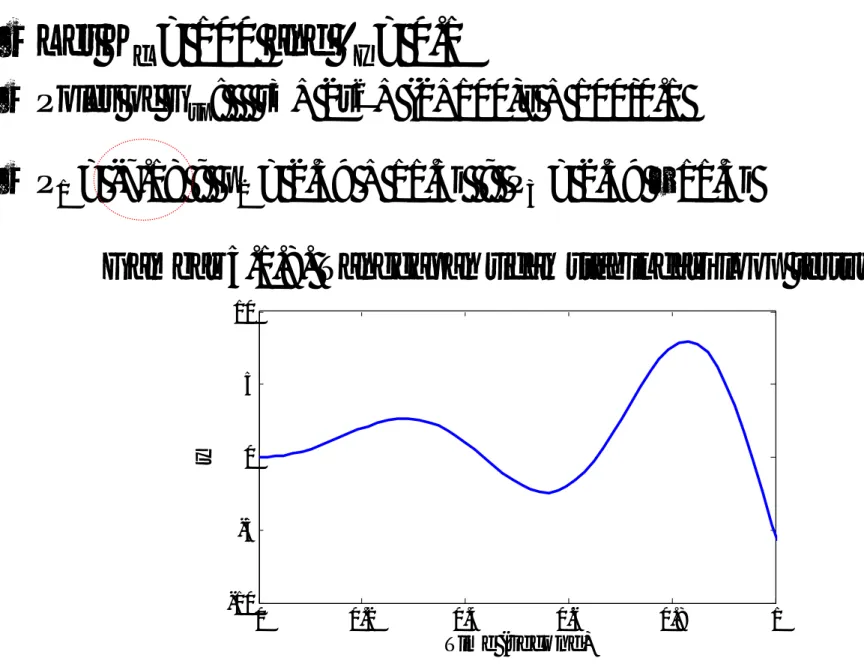
Analisis Kestabilan Sistem Pengendalian Umpan Balik
Teks penuh
Gambar

Dokumen terkait
BEBERAPA HARI LALU DIADAKAN KEGIATAN UNTUK ANAK-ANAK BERUPA OLAHRAGA.
Acquired artinya didapat, bukan penyakit keturunan; Immuno berarti sistem kekebalan tubuh, Deficiency artinya kekurangan; sedangkan Syndrome adalah kumpulan gejala.Orang
PEKERJAAN UMUM (PU) / SEBAGAI KOTA TERBAIK PERTAMA / DI BIDANG PENANGANAN PEMUKIMAN KUMUH KATEGORI KOTA BESAR // HAL TERSEBUT SEPERTI YANG DISAMPAIKAN HERRY ZUDIANTO..
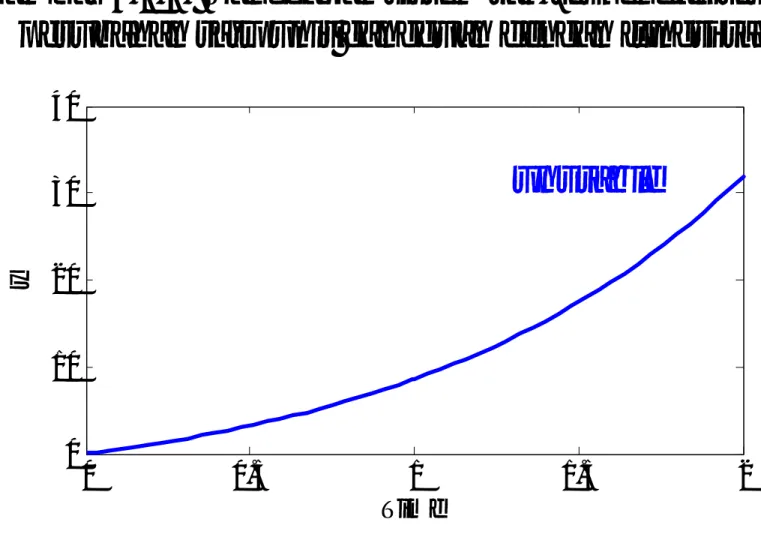
Tabel 4.13 Resume Hasil Uji Pengaruh Abu Vulkanik Terhadap Kuat Geser Error!. Bookmark
100 Pertanyaan Seputar HIV/AIDS Yang Perlu Anda Ketahui, Medan: USU Press. 111 Pertanyaan Seputar HIV/AIDS Yang Perlu Anda Ketahui,Medan:
Didukung oleh penelitian [9] pada pertumbuhan pucuk tomat dari hipokotil juga menunjukkan hasil yang lebih baik pada kontrol, dibandingkan dengan perlakuan lainnya
Berdasarkan data di atas terlihat bahwa terdapat perbedaan hasil yang diperoleh untuk air laut lebih tinggi dibandingkan dengan sedimen dimana yang seharusnya kandungan logam
Pengendalian secara kimia dengan bakterisida terhadap penyakit bakteri pada tanaman kedelai tidak dianjurkan mengingat mahalnya biaya yang diperlukan