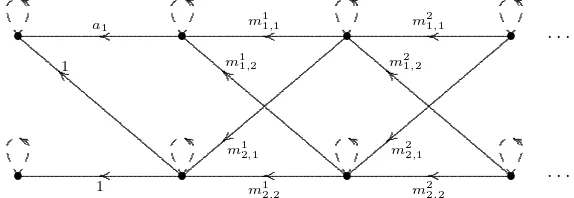
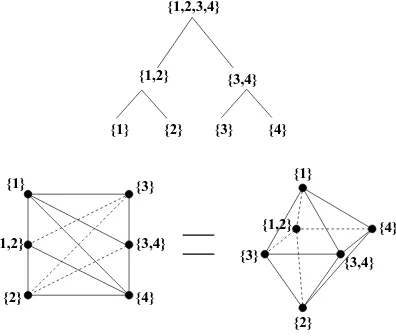
Documenta Mathematica
Journal der
Deutschen Mathematiker-Vereinigung
Gegr¨
undet 1996
{1}
{4} {3,4}
{2} {3}
{1,2}
{1} {3}
{3,4}
{4} {2}
{1,2}
{1,2,3,4}
{2}
{3,4} {1,2}
{1} {3} {4}
ver¨offentlicht Forschungsarbeiten aus allen mathematischen Gebieten und wird in traditioneller Weise referiert. Es wird indiziert durch Mathematical Reviews, Science Citation Index Expanded, Zentralblatt f¨ur Mathematik.
Artikel k¨onnen als TEX-Dateien per E-Mail bei einem der Herausgeber eingereicht werden. Hinweise f¨ur die Vorbereitung der Artikel k¨onnen unter der unten angegebe-nen WWW-Adresse gefunden werden.
Documenta Mathematica, Journal der Deutschen Mathematiker-Vereinigung, publishes research manuscripts out of all mathematical fields and is refereed in the traditional manner. It is indexed in Mathematical Reviews, Science Citation Index Expanded, Zentralblatt f¨ur Mathematik.
Manuscripts should be submitted as TEX -files by e-mail to one of the editors. Hints for manuscript preparation can be found under the following web address.
http://www.math.uni-bielefeld.de/documenta
Gesch¨aftsf¨uhrende Herausgeber / Managing Editors: Alfred K. Louis, Saarbr¨ucken louis@num.uni-sb.de
Ulf Rehmann (techn.), Bielefeld rehmann@math.uni-bielefeld.de Peter Schneider, M¨unster pschnei@math.uni-muenster.de Herausgeber / Editors:
Don Blasius, Los Angeles blasius@math.ucla.edu Joachim Cuntz, M¨unster cuntz@math.uni-muenster.de Patrick Delorme, Marseille delorme@iml.univ-mrs.fr Gavril Farkas, Berlin (HU) farkas@math.hu-berlin.de Edward Frenkel, Berkeley frenkel@math.berkeley.edu Friedrich G¨otze, Bielefeld goetze@math.uni-bielefeld.de Ursula Hamenst¨adt, Bonn ursula@math.uni-bonn.de Lars Hesselholt, Cambridge, MA (MIT) larsh@math.mit.edu Max Karoubi, Paris karoubi@math.jussieu.fr
Stephen Lichtenbaum Stephen Lichtenbaum@brown.edu Eckhard Meinrenken, Toronto mein@math.toronto.edu
Alexander S. Merkurjev, Los Angeles merkurev@math.ucla.edu Anil Nerode, Ithaca anil@math.cornell.edu
Thomas Peternell, Bayreuth Thomas.Peternell@uni-bayreuth.de Eric Todd Quinto, Medford Todd.Quinto@tufts.edu
Takeshi Saito, Tokyo t-saito@ms.u-tokyo.ac.jp Stefan Schwede, Bonn schwede@math.uni-bonn.de Heinz Siedentop, M¨unchen (LMU) h.s@lmu.de
Wolfgang Soergel, Freiburg soergel@mathematik.uni-freiburg.de G¨unter M. Ziegler, Berlin (TU) ziegler@math.tu-berlin.de
ISSN 1431-0635 (Print), ISSN 1431-0643 (Internet)
SPARC
Leading Edge
Band 13, 2008 V. Gritsenko, K. Hulek and G. K. Sankaran
Hirzebruch-Mumford Proportionality and Locally Symmetric Varieties of Orthogonal
Type 1–19
Tom Leinster
The Euler Characteristic of a Category 21–49 Sergey Morozov
Essential Spectrum of Multiparticle
Brown–Ravenhall Operators in External Field 51–79 A. Vishik, K. Zainoulline
Motivic Splitting Lemma 81–96
J¨org Winkelmann
On Tameness and Growth Conditions 97–101 V. Bach, J. Hoppe, D. Lundholm
Dynamical Symmetries in
Supersymmetric Matrix 103–116
J¨urgen Ritter and Alfred Weiss
Equivariant Iwasawa Theory: An Example 117–129 Guido Kings
Degeneration of Polylogarithms and Special Values
ofL-Functions for Totally Real Fields 131–159 Alex Kumjian, David Pask, Aidan Sims
C∗-Algebras Associated to Coverings of k-Graphs 161–205 Alex Postnikov, Victor Reiner, Lauren Williams
Faces of Generalized Permutohedra 207–273 Denis Perrot
Secondary Invariants for Frechet Algebras
and Quasihomomorphisms 275–363
Paul S. Muhly and Baruch Solel Schur Class Operator Functions
and Automorphisms of Hardy Algebras 365–411 Viorel Costeanu
On the 2-Typical De Rham-Witt Complex 413–452 Ciro Ciliberto, Gerard van der Geer
K-Theory of Log-Schemes I 505–551 Eric A. Carlen and Elliott H. Lieb
Brascamp-Lieb Inequalities
for Non-Commutative Integration 553–584 Gereon Quick
Profinite Homotopy Theory 585–612
F. D´eglise
Around the Gysin Triangle II. 613–675
Matthias K¨unzer
Comparison of Spectral Sequences
Involving Bifunctors 677–737
Goro Shimura
Arithmetic of Hermitian Forms 739–774
Goro Shimura
The Critical Values of Certain Dirichlet Series 775–794 Christian Ausoni, Bjørn Ian Dundas and John Rognes
Divisibility of the Dirac Magnetic Monopole
as a Two-Vector Bundle over the Three-Sphere 795–801 Timothy Logvinenko
Natural G-Constellation Families 803–823 Eva Viehmann
The Global Structure of Moduli Spaces
Hirzebruch-Mumford Proportionality and
Locally Symmetric Varieties of Orthogonal
Type
V. Gritsenko, K. Hulek and G. K. Sankaran
Received: September 28, 2006 Revised: February 17, 2008
Communicated by Thomas Peternell
Abstract. For many classical moduli spaces of orthogonal type there are results about the Kodaira dimension. But nothing is known in the case of dimension greater than 19. In this paper we obtain the first results in this direction. In particular the modular variety defined by the orthogonal group of the even unimodular lattice of signature (2,8m+ 2) is of general type ifm≥5.
2000 Mathematics Subject Classification: 14J15, 11F55
Keywords and Phrases: Locally symmetric variety; modular form; Hirzebruch-Mumford proportionality
1 Modular varieties of orthogonal type
LetLbe an integral indefinite lattice of signature (2, n) and (,) the associated bilinear form. By DL we denote a connected component of the homogeneous
type IV complex domain of dimensionn
DL={[w]∈P(L⊗C)|(w, w) = 0, (w, w)>0}+.
O+(L) is the index 2 subgroup of the integral orthogonal group O(L) that leaves DL invariant. Any subgroup Γ of O+(L) of finite index determines a
modular variety
FL(Γ) = Γ\ DL.
For some special lattices L and subgroups Γ < O+(L) one obtains in this way the moduli spaces of polarised abelian or Kummer surfaces (n = 3, see [GH]), the moduli space of Enriques surfaces (n = 10, see [BHPV]), and the moduli spaces of polarised or lattice-polarised K3 surfaces (0 < n ≤ 19, see [Nik1, Dol]). Other interesting modular varieties of orthogonal type include the period domains of irreducible symplectic manifolds: see [GHS3].
It is natural to ask about the birational type of FL(Γ). For many classical
moduli spaces of orthogonal type there are results about the Kodaira dimension, but nothing is known in the case of dimension greater than 19. In this paper we obtain the first results in this direction. We determine the Kodaira dimension of many quasi-projective varieties associated with two series of even lattices. To explain what these varieties are, we first introduce the stable orthogonal group O(L) of a nondegenerate even latticee L. This is defined (see [Nik2] for more details) to be the subgroup of O(L) which acts trivially on the discrim-inant group AL =L∨/L, where L∨ is the dual lattice. If Γ<O(L) then we
write eΓ = Γ∩O(L). Note that ife Lis unimodular then O(L) = O(L).e
The first series of varieties we want to study, which we call the modular varieties ofunimodular type, is
FII(m)= O
+(II
2,8m+2)\DII2,8m+2. (1)
FII(m) is of dimension 8m+ 2 and arises from the even unimodular lattice of
signature (2,8m+ 2)
II2,8m+2= 2U⊕mE8(−1),
where U denotes the hyperbolic plane and E8(−1) is the negative definite lattice associated to the root system E8. The varietyFII(2) is the moduli space
of elliptically fibred K3 surfaces with a section (see e.g. [CM, Section 2]). The casem= 3 is of particular interest: it arises in the context of the fake Monster Lie algebra [B1].
The second series, which we call the modular varieties ofK3 type, is
F2(md)=Oe
+
(L(2md))\DL(m)
2d . (2)
F2(md)is of dimension 8m+ 3 and arises from the lattice
L(2md)= 2U⊕mE8(−1)⊕ h−2di,
whereh−2didenotes a lattice generated by a vector of square−2d.
The first three members of the series F2(md) have interpretations as moduli spaces. F2(2)d is the moduli space of polarisedK3 surfaces of degree 2d. For m= 1 the 11-dimensional varietyF2(1)d is the moduli space of lattice-polarised K3 surfaces, where the polarisation is defined by the hyperbolic lattice h2di ⊕ E8(−1) (see [Nik1, Dol]). Form= 0 anddprime the 3-foldF2(0)d is the moduli
Theorem 1.1 The modular varieties of unimodular andK3type are varieties of general type ifmanddare sufficiently large. More precisely:
(i) If m≥5 then the modular varietiesFII(m) andF2(md)(for anyd≥1) are of general type.
(ii) Form= 4the varietiesF2(4)d are of general type ifd≥3and d6= 4. (iii) Form= 3the varietiesF2(3)d are of general type ifd≥1346. (iv) Form= 1the varietiesF2(1)d are of general type ifd≥1537488.
Remark. The methods of this paper are also applicable ifm= 2. Using them, one can show that the moduli spaceF2(2)d of polarised K3 surfaces of degree 2d
is of general type ifd≥231000. This case was studied in [GHS2], where, using a different method involving special pull-backs of the Borcherds automorphic form Φ12on the domainDII2,26, we proved thatF2(2)d is of general type ifd >61
or d= 46, 50, 54, 57, 58, 60.
The methods of [GHS2] do not appear to be applicable in the other cases studied here. Instead, the proof of Theorem 1.1 depends on the existence of a good toroidal compactification ofFL(Γ), which was proved in [GHS2], and on
the exact formula for the Hirzebruch-Mumford volume of the orthogonal group found in [GHS1].
We shall construct pluricanonical forms on a suitable compactification of the modular variety FL(Γ) by means of modular forms. Let Γ < O+(L) be a
subgroup of finite index, which naturally acts on the affine coneD•
L over DL.
In what follows we assume that dimDL≥3.
Definition 1.2 A modular form of weightk and characterχ: Γ →C∗ with
respect to the groupΓis a holomorphic function
F :D•
L→C which has the two properties
F(tz) = t−kF(z)
∀t∈C∗,
F(g(z)) = χ(g)F(z) ∀g∈Γ.
The space of modular forms is denoted byMk(Γ, χ). The space of cusp forms,
i.e. modular forms vanishing on the boundary of the Baily–Borel compactifi-cation of Γ\DL, is denoted by Sk(Γ, χ). We can reformulate the definition of
modular forms in geometric terms. LetF ∈Mkn(Γ,detk) be a modular form,
wherenis the dimension ofDL. Then
F(dZ)k ∈H0(FL(Γ)◦,Ω⊗k),
where dZ is a holomorphic volume form on DL, Ω is the sheaf of germs of
canonical n-forms on FL(Γ) and FL(Γ)◦ is the open smooth part of FL(Γ)
The main question in the proof of Theorem 1.1 is how to extend the form F(dZ)k to
FL(Γ) and to a suitable toroidal compactificationFL(Γ)tor. There
are three possible kinds of obstruction to this, which we call (as in [GHS2]) elliptic, reflective and cusp obstructions. Elliptic obstructions arise ifFL(Γ)tor
has non-canonical singularities arising from fixed loci of the action of the group Γ. Reflective obstructions arise because the projection π is branched along divisors whose general point is smooth inFL(Γ). Cusp obstructions arise when
we extend the form fromFL(Γ) toFL(Γ)tor.
The problem of elliptic obstructions was solved forn≥9 in [GHS2].
Theorem 1.3 ([GHS2, Theorem 2.1]) Let L be a lattice of signature (2, n)
withn≥9, and letΓ<O+(L)be a subgroup of finite index. Then there exists a toroidal compactificationFL(Γ)tor ofFL(Γ) = Γ\DLsuch thatFL(Γ)tor has canonical singularities and there are no branch divisors in the boundary. The branch divisors inFL(Γ)arise from the fixed divisors of reflections.
Reflective obstructions, that is branch divisors, are a special problem related to the orthogonal group. They do not appear in the case of moduli spaces of polarised abelian varieties of dimension greater than 2, where the modular group is the symplectic group. There are no quasi-reflections in the symplectic group even for g= 3.
The branch divisor is defined by special reflective vectors in the latticeL. This description is given in §2. To estimate the reflective obstructions we use the Hirzebruch-Mumford proportionality principle and the exact formula for the Hirzebruch-Mumford volume of the orthogonal group found in [GHS1]. We do the numerical estimation in§4.
We treat the cusp obstructions in §3, using special cusp forms of low weight (the lifting of Jacobi forms) constructed in [G2] and the low-weight cusp form trick (see [G2] and [GHS2]).
2 The branch divisors
To estimate the obstruction to extending pluricanonical forms to a smooth projective model of FL(Oe
+
(L)) we have to determine the branch divisors of the projection
π:DL→ FL(Oe
+
(L)) =Oe+(L)\ DL. (3)
According to [GHS2, Corollary 2.13] these divisors are defined by reflections σr∈O+(L), where
σr(l) =l−2(l, r)
(r, r)r,
coming from vectorsr ∈L with r2 <0 that arestably reflective: by this we mean thatris primitive andσr or−σris inOe
+
(L). By a (k)-vector fork∈Z
Let D be the exponent of the finite abelian group AL and let the divisor
div(r) of r ∈ L be the positive generator of the ideal (l, L). We note that r∗ =r/div(r) is a primitive vector in L∨. In [GHS2, Propositions 3.1–3.2] we
proved the following.
Lemma 2.1 LetLbe an even integral lattice of signature(2, n). Ifσr∈Oe
+ (L)
thenr2 =−2. If−σ
r∈Oe
+
(L), then r2=−2D anddiv(r) =D≡1 mod2or r2=−Dand div(r) =D orD/2.
We need also the following well-known property of the stable orthogonal group.
Lemma 2.2 For any sublatticeM of an even latticeLthe groupO(Me )can be considered as a subgroup ofO(L)e .
Proof. LetM⊥ be the orthogonal complement ofM in L. We have as usual
M⊕M⊥⊂L⊂L∨⊂M∨⊕(M⊥)∨.
We can extendg ∈O(Me ) to M ⊕M⊥ by putting g|
M⊥ ≡id. It is clear that
g ∈ O(Me ⊕M⊥). For any l∨ ∈ L∨ we have g(l∨) ∈ l∨+ (M ⊕M⊥). In
particular,g(l)∈Lfor anyl∈Landg∈O(L).e ✷ We can describe the components of the branch locus in terms of homogeneous domains. Forra stably reflective vector inLwe put
Hr={[w]∈P(L⊗C)|(w, r) = 0},
and let N be the union of all hyperplane sections Hr∩ DL over all stably
reflective vectorsr.
Proposition 2.3 Letr∈Lbe a stably reflective vector: suppose thatrand
L do not satisfy D = 4, r2 =
−4, div(r) = 2. Let Kr be the orthogonal complement of r in L. Then the associated component π(Hr∩ DL) of the branch locusN is of the formOe+(Kr)\DKr.
Proof. We haveHr∩ DL=P(Kr)∩ DL=DKr. Let
ΓKr ={ϕ∈Oe
+
(L)|ϕ(Kr) =Kr}. (4)
ΓKr maps to a subgroup of O+(Kr). The inclusion of O(Ke r) in O(L)e
(Lemma 2.2) preserves the spinor norm (see [GHS1, §3.1]), because Kr has
signature (2, n−1) and soOe+(Kr) becomes a subgroup ofOe
+ (L). Therefore the image of ΓKr containsOe
+
(Kr) for any r. Now we prove that
this image coincides with Oe+(Kr) for allr, except perhaps ifD = 4,r2=−4
Let us consider the inclusions
hri ⊕Kr⊂L⊂L∨⊂ hri∨⊕Kr∨.
By standard arguments (see [GHS2, Proposition 3.6]) we see that
|detKr|=|detL| · |r
2|
div(r)2 and [L:hri ⊕Kr] =
|r2|
div(r) = 1 or 2.
If the index is 1, then it is clear that the image of ΓKr is Oe
+
(Kr). Let us
assume that the index is equal to 2. In this case the lattice hri∨ is generated byr∨=−r/(r, r) =r∗/2, wherer∗=r/div(r) is a primitive vector inL∨. In
particular r∨ represents a non-trivial class in hri∨
⊕K∨
r modulo L∨. Let us
takek∨∈K∨
r such thatk∨6∈L∨. Thenk∨+r∨∈L∨ and
ϕ(k∨)−k∨≡r∨−ϕ(r∨) modL. We note that ifϕ∈ΓKr thenϕ(r) =±r. Hence
ϕ(k∨)−k∨≡
(
0 modL ifϕ(r) =r r∗ modL ifϕ(r) =−r
Since ϕ(r∗)≡r∗ modL, we cannot have ϕ(r) = −r unless div(r) = 1 or 2.
Therefore we have proved that ϕ(k∨)≡k∨ modK
r (Kr =Kr∨∩L), except
possibly ifD= 4, r2=
−4, div(r) = 2. ✷
The groupOe+(L) acts onN. We need to estimate the number of components of Oe+(L)\ N. This will enable us to estimate the reflective obstructions to extending pluricanonical forms which arise from these branch loci.
For the even unimodular latticeII2,8m+2any primitive vectorrhas div(r) = 1. Consequentlyris stably reflective if and only ifr2=
−2.
For L(2md) the reflections and the corresponding branch divisors arise in two different ways, according to Lemma 2.1. We shall classify the orbits of such vectors.
Proposition 2.4 Supposedis a positive integer.
(i) Any two (−2)-vectors in the lattice II2,8m+2 are equivalent modulo O+(II2,8m+2), and the orthogonal complement of a(−2)-vectorr is
iso-metric to
KII(m)=U⊕mE8(−1)⊕ h2i.
(ii) There is oneOe+(L(2md))-orbit of(−2)-vectorsrinL(2md)withdiv(r) = 1. If
d≡1mod4then there is a second orbit of(−2)-vectors, withdiv(r) = 2. The orthogonal complement of a(−2)-vectorrinL(2md)is isometric to
ifdiv(r) = 1, and to
N2(md)=U⊕mE8(−1)⊕
1 2
1−d
2 1
,
ifdiv(r) = 2.
(iii) The orthogonal complement of a(−2d)-vectorrin L(2md) is isometric to
II2,8m+2= 2U⊕mE8(−1)
ifdiv(r) = 2d, and to
K2(m)=U⊕mE8(−1)⊕ h2i⊕ h−2i or T2,8m+2=U⊕U(2)⊕mE8(−1)
ifdiv(r) =d.
(iv) Suppose d > 1. The number of O(Le (2md))-orbits of (−2d)-vectors with
div(r) = 2dis2ρ(d). The number ofO(Le (m)
2d )-orbits of(−2d)-vectors with
div(r) =dis
2ρ(d) ifdis odd or d≡4 mod 8; 2ρ(d)+1 if d
≡0 mod 8; 2ρ(d)−1 if d≡2 mod 4.
Hereρ(d)is the number of prime divisors ofd.
Proof. If the lattice L contains two hyperbolic planes then according to the well-known result of Eichler (see [E,§10]) theOe+(L)-orbit of a primitive vector l ∈ L is completely defined by two invariants: by its length (l, l) and by its imagel∗+Lin the discriminant groupA
L, wherel∗=l/div(l).
i) Ifuis a primitive vector of an even unimodular latticeII2,8m+2then div(u) = 1 and there is only one O(II2,8m+2)-orbit of (−2)-vectors. Therefore we can takerto be a (−2)-vector inU, and the form of the orthogonal complement is obvious.
ii) In the lattice L(2md) we fix a generator h of its h−2di-part. Then for any r∈L(2md)we can write r=u+xh, whereu∈II2,8m+2 and x∈Z. It is clear that div(r) dividesr2. If f|div(r), wheref = 2, d or 2d, then the vector u is also divisible by f. Therefore the (−2)-vectors form two possible orbits of vectors with divisor equal to 1 or 2. Ifr2 =−2 and div(r) = 2 then u= 2u
0 withu0∈2U⊕mE8(−1) and we see that in this cased≡1 mod 4. This gives us two different orbits for such d. In both cases we can find a (−2)-vector r in the sublattice U⊕ h−2di. Elementary calculation gives us the orthogonal complement ofr.
iv) To find the number of orbits of (−2d)-vectors we have to consider two cases. a) Let div(r) = 2d. Thenr= 2du+xh andr∗ ≡(x/2d)h modL, whereu∈
II2,8m+2andxis modulo 2d. Moreover (r, r) = 4d2(u, u)−x22d=−2d. Thus x2
≡1 mod 4d. This congruence has 2ρ(d)solutions modulo 2d. For any such x mod 2dwe can find a vectoruin 2U⊕mE8(−1) with (u, u) = (x2−1)/2d. Thenr= 2du+xhis primitive (becauseuis not divisible by any divisor ofx) and (r, r) =−2d.
b) Let div(r) =d. Thenr=du+xh, whereuis primitive,r∗≡(x/d)h modL and xis modulo d. We have (r∗, r∗)≡ −2x2/d mod 2Z andx2 ≡1 mod d. For any solution modulodwe can find as aboveu∈2U⊕mE8(−1) such that r=du+xhis primitive and (r, r) =−2d. It is easy to see that the number of solutions{x modd|x2≡1 modd} is as stated. ✷
Remark. To calculate the number of the branch divisors arising from vectors r with r2 =
−2done has to divide the corresponding number of orbits found in Proposition 2.4(iv) by 2 if d > 2. This is because ±r determine different orbits but the same branch divisor. For d= 2 the proof shows that there is one divisor for each orbit given in Proposition 2.4(iv).
3 Modular forms of low weight
In this section we let L= 2U⊕L0 be an even lattice of signature (2, n) with two hyperbolic planes. We choose a primitive isotropic vector c1 in L. This vector determines a 0-dimensional cusp and a tube realisation of the domain
DL. The tube domain (see the definition ofH(L1) below) is a complexification of the positive cone of the hyperbolic latticeL1=c⊥1/c1. If div(c1) = 1 we call this cuspstandard (as above, by [E] there is only one standard cusp). In this caseL1=U⊕L0. In [GHS2,§4] we proved that any 1-dimensional boundary component of Oe+(L)\ DL contains the standard 0-dimensional cusp if every
isotropic (with respect to the discriminant form: see [Nik2, §1.3]) subgroup of AL is cyclic.
Let us fix a 1-dimensional cusp by choosing two copies of U in L. (One has to add to c1 a primitive isotropic vector c2 ∈ L1 with div(c2) = 1). Then L=U ⊕L1=U⊕(U⊕L0) and the construction of the tube domain may be written down simply in coordinates. We have
H(L1) =Hn={Z = (zn, . . . , z1)∈H1×Cn−2×H1; (ImZ,ImZ)L1 >0},
where Z∈L1⊗Cand (zn−1, . . . , z2)∈L0⊗C. (We representZ as a column vector.) An isomorphism betweenHn andDL is given by
p:Hn −→ DL (5)
Z = (zn, . . . , z1) 7−→ − 1
2(Z, Z)L1 :zn :· · ·:z1: 1
.
The action of O+(L⊗R) onHnis given by the usual fractional linear
defined byg∈O+(L⊗R) is equal to det(g)j(g, Z)−n, wherej(g, Z) is the last
((n+ 2)-nd) coordinate ofg p(Z)∈ DL. Using this we define the automorphic
factor
J: O+(L⊗R)× Hn+2 → C∗ (g, Z) 7→ (detg)−1
·j(g, Z)n.
The connection with pluricanonical forms is the following. Consider the form
dZ=dz1∧ · · · ∧dzn∈Ωn(Hn).
F(dZ)k is a Γ-invariant k-fold pluricanonical form on H
n, for Γ a subgroup
of finite index of O+(L), ifF g(Z)=J(g, Z)kF(Z) for anyg ∈Γ; in other
words ifF ∈Mnk(Γ,detk) (see Definition 1.2). To prove Theorem 1.1 we need
cusp forms of weight smaller than the dimension of the corresponding modular variety.
Proposition 3.1 For unimodular type, cusp forms of weight12 + 4m exist: that is
dimS12+4m(O+(II2,8m+2))>0.
ForK3type we have the bounds
dimS11+4m(Oe
+
(L(2md))) > 0 if d >1; dimS10+4m(Oe
+
(L(2md))) > 0 if d≥1; dimS7+4m(Oe
+
(L(2md))) > 0 if d≥4; dimS6+4m(Oe
+
(L(2md))) > 0 if d= 3 or d≥5; dimS5+4m(Oe
+
(L(2md))) > 0 if d= 5 or d≥7;
dimS2+4m(Oe
+
(L(2md))) > 0 if d >180.
Proof. For anyF(Z)∈Mk(Oe
+
(L)) we can consider its Fourier-Jacobi expan-sion at the 1-dimenexpan-sional cusp fixed above
F(Z) =f0(z1) +
X
m≥1
fm(z1;z2, . . . zn−1) exp(2πimzn).
A lifting construction of modular formsF(Z)∈Mk(Oe
+
The dimension ofJk,1(L0) depends only on the discriminant form and the rank ofL0(see [G2, Lemma 2.4]). In particular, for the special cases ofL=II2,8m+2 andL=L(2md)we have
Jkcusp+4m,1(mE8(−1))∼=Sk(SL2(Z)) and
Jkcusp+4m,1(mE8(−1)⊕ h−2di)∼=Jk,dcusp,
where Jk,dcusp is the space of the usual Jacobi cusp forms in two variables of weightkand indexd(see [EZ]) andSk(SL2(Z)) is the space of weightkcusp forms for SL2(Z).
The lifting of a Jacobi cusp form of index one is a cusp form of the same weight with respect to O+(II2,8m+2) or Oe
+
(L(2md)) with trivial character. The fact that we get a cusp form was proved in [G2] for maximal lattices, i.e., if dis square-free. In [GHS2, §4] we extended this to all lattices L for which the isotropic subgroups of the discriminant AL are all cyclic, which is true in all
cases considered here.
To prove the unimodular type case of Proposition 3.1 we can take the Jacobi form corresponding to the cusp form ∆12(τ). Using the Jacobi lifting construc-tion we obtain a cusp form of weight 12 + 4mwith respect to O+(II2,8m+2). For the K3 type case we need the dimension formula for the space of Jacobi cusp formsJk,dcusp (see [EZ]). For a positive integerl one sets
{l}12=
⌊ l
12⌋ ifl6≡2 mod 12
⌊l
12⌋ −1 ifl≡2 mod 12. Then ifk >2 is even
dimJk,dcusp= d X
j=0
{k+ 2j}12−
j2 4d
,
and ifkis odd
dimJk,dcusp=
d−1
X
j=1
{k−1 + 2j}12−
j2 4d
.
This gives the bounds claimed. For k = 2, using the results of [SZ] one can also calculate dimJ2cusp,d : there is an extra term,⌈σ0(d)/2⌉, whereσ0(d) denotes the number of divisors ofd. This gives dimJ2cusp,d >0 ifd >180 and for some
smaller values ofd. ✷
4 Kodaira dimension results
particular all singularities are canonical and there is no ramification divisor which is contained in the boundary. Then the canonical divisor (as aQ-divisor) is given by KFL(Γ)tor =nM −V −D whereM is the line bundle of modular
forms of weight 1,nis the dimension ofFL(Γ),V is the branch locus (which is
given by reflections) andDis the boundary. Hence in order to constructk-fold pluricanonical forms we must find modular forms of weight kn which vanish of orderkalong the branch divisor and the boundary. This also suffices since
FL(Γ)tor has canonical singularities.
Our strategy is the following. For Γ⊆Oe+(L) we choose a cusp formFa ∈Sa(Γ)
of low weight a, i.e. astrictly less than the dimension. Then we consider ele-mentsF ∈Fk
aMk(n−a)(Γ,detk): for simplicity we assume thatkis even. Such anF vanishes to order at least kon the boundary of any toroidal compactifi-cation. Hence if dZ is the volume element onDL defined in§3 it follows that
F(dZ)k extends as a k-fold pluricanonical form to the general point of every
boundary component ofFL(Γ)tor. Since we have chosen the toroidal
compact-ification so that all singularities are canonical and that there is no ramcompact-ification divisor which is contained in the boundary the only obstructions to extending F(dZ)k to a smooth projective model are the reflective obstructions, coming
from the ramification divisor of the quotient map π: DL → FL(Γ) studied in
§2.
LetDK be an irreducible component of this ramification divisor. Recall from
Proposition 2.3 thatDK =P(K⊗C)∩ DL where K =Kr is the orthogonal
complement of a stably reflective vector r. For the lattices chosen in The-orem 1.1 all irreducible components of the ramification divisor are given in Proposition 2.4.
Proposition 4.1 We assume that k is even and that the dimensionn ≥ 9. For Γ ⊆ Oe+(L), the obstruction to extending forms F(dZ)k where F
∈
Fk
aMk(n−a)(Γ)toFL(Γ)tor lies in the space
B=M
K
B(K) =M
K k/2−1
M
ν=0
Mk(n−a)+2ν(Γ∩Oe
+ (K)),
where the direct sum is taken over all irreducible componentsDK of the rami-fication divisor of the quotient mapπ: DL→ F(Γ).
Proof. Letσ∈Γ be plus or minus a reflection whose fixed point locus isDK.
We can extend the differential form provided thatF vanishes of orderk along every irreducible componentDK of the ramification divisor.
IfFa vanishes alongDK thenK gives no restriction on the second factor of the
modular formF.
Now let {w = 0} be a local equation for DK. Then σ∗(w) = −w (this is
independent of whether σ or −σ is the reflection). For every modular form F ∈ Mk(Γ) of even weight we have F σ(z) = F(z). This implies that if
We denote byM2b(Γ)(−νDK) the space of modular forms of weight 2b which
vanish of order at least ν along DK. Since the weight is even we have
M2b(Γ)(−DK) = M2b(Γ)(−2DK). For F ∈ M2b(Γ)(−2νDK) we consider
(F/w2ν) as a function on
DK. From the definition of modular form
(Def-inition 1.2) it follows that this function is holomorphic, Γ∩ ΓK-invariant
(see equation (4)) and homogeneous of degree 2b+ 2ν. Thus (F/w2ν)
|DK ∈
M2(b+ν)(Γ∩ΓK). In Proposition 2.3 we saw that, ΓK containsOe
+
(K) as sub-group of Oe+(L)(with equality in almost all cases), so we may replace Γ∩ΓK
by Γ∩Oe+(K). In this way we obtain an exact sequence
0→M2b(Γ)(−(2 + 2ν)DK)→M2b(Γ)(−2νDK)→M2(b+ν)(Γ∩Oe +
(K)),
where the last map is given by F7→F/w2ν. This gives the result. ✷
Now we proceed with the proof of Theorem 1.1.
Let L be a lattice of signature (2, n) and Γ <Oe+(L): recall that k is even. According to Proposition 4.1 we can find pluricanonical differential forms on
FL(Γ)tor if
CB(Γ) = dimMk(n−a)(Γ)−
X
K
dimB(K)>0, (6)
where summation is taken over all irreducible components of the ramification divisor (see the remark at the end of §2). It now remains to estimate the dimension ofB(K) for each of the finitely many components of the ramification locus in the cases we are interested in, namely Γ = O+(II2,8m+2) and Γ =
e
O+(L(2md)).
According to the Hirzebruch-Mumford proportionality principle
dimMk(Γ) =
2
n!volHM(Γ)k
n+O(kn−1).
The exact formula for the Hirzebruch-Mumford volume volHM for any
indef-inite orthogonal group was obtained in [GHS1]. It depends mainly on the determinant and on the local densities of the lattice L. Here we simply quote the estimates of the dimensions of certain spaces of cusp forms.
The case of II2,8m+2 is easier because the branch divisor has only one irre-ducible component defined by any (−2)-vectorr. According to Proposition 2.4 the orthogonal complement Kr is KII(m). This lattice differs from the lattice
L(2m), whose Hirzebruch-Mumford volume was calculated in [GHS1,§3.5], only by one copy of the hyperbolic plane. Therefore
volHMOe
+
(L(2m)) = (B8m+4/(8m+ 4)) volHMOe
+
(KII(m)), and hence, for evenk,
dimMk(Oe
+
(KII(m))) =
21−4m
(8m+ 1)!·
B2. . . B8m+2 (8m+ 2)!! k
where the Bi are Bernoulli numbers. Assume thatm≥3. Let us take a cusp
form
F ∈S4m+12(O+(II2,8m+2))
from Proposition 3.1. In this case the dimension of the obstruction spaceB of Proposition 4.1 for the pluricanonical forms of orderk= 2k1is given by
k1X−1
In [GHS1, §3.3] we computed the leading term of the dimension of the space of modular forms for O+(II2,8m+2). Comparing these two we see that the
Using this estimate we easily obtain that (7) holds ifm≥5. Therefore we have proved Theorem 1.1 for the latticeII2,8m+2.
Next we consider the latticeL(2md)of K3 type. For this lattice the branch divisor ofF2(md)is calculated in Proposition 2.4. It contains one or two (ifd≡1 mod 4)
components defined by (−2)-vectors and some number of components defined by (−2d)-vectors. To estimate the obstruction constantCB(Γ) in (6) we use the
dimension formulae for the space of modular forms with respect to the group
e
O+(M), where M is one of the following lattices from Proposition 2.4: L(2md) (the main group);K2(md)andN2(md)(the (−2)-obstruction);M2,8m+2,K2(m)and T2,8m+2 (the (−2d)-obstruction). The corresponding dimension formulae were found in [GHS1] (see §§3.5, 3.6.1–3.6.2, 3.3 and 3.4). The branch divisor of (−2d)-type appears only ifd >1. We note that Therefore in order to estimateCB(Γ) we can assume that all (−2d)-divisors
where
B(−2)= dimB(K2(md)) + dimB(N
(m) 2d ),
B(−2d)= 2ρ(d)−1(dimB(M2,8m+2) + 2hddimB(T2,8m+2))
andB(K) is the obstruction space from Proposition 4.1. Byhd we denote the
sumδ0,d(8)−δ2,d(4), where d(n) isdmodnand δ is the Kronecker delta (see Proposition 2.4 and the remark following it).
For any lattice considered above
All terms in (10) contain a common factor. First
dimM2k1w(Oe From [GHS1, (16)] it follows that
volHM(Oe
(see [GHS1, 3.6.2]). It follows that
where
Hereχ0,f denotes the principal Dirichlet character modulof.
We note that |PK(n)|<1 and|PN(n)|<1 for anyd. We conclude that
B(−2)< Cm,dk1,wEw(8m+ 3)b(−2)
whereb(−2)= 28m+3−1.
The (−2d)-contribution is calculated according to [GHS1, 3.3–3.4]. We note that Oe+(T2,8m+2) is a subgroup ofOe
As a result we see that that the obstruction constantCB(Oe
For m ≤ 3 we choose Fa of weight 4m+ 2, i.e. we take w = 4m+ 1. Such
a cusp form exists ifd >180 according to Proposition 3.1. For such dwe see that β(−2d)<1. Then the obstruction constantCB(Oe
+
(L(2md))) is positive if
BB48mm+2+2
(1 +4m1+ 1)8m+3(28m+3+ 2)<
√
d.
This inequality gives us the bound ondin Theorem 1.1. This completes the proof of Theorem 1.1.
In the proof of Theorem 1.1 above we have seen that the (−2)-part of the branch divisor forms the most important reflective obstruction to the extension of the
e
O+(L(2md))-invariant differential forms to a smooth compact model ofF2(md). Let
us consider the double covering SF(2md) of F2(md) for d >1 determined by the special orthogonal group:
SF(2md)=SOf
+
(L(2md))\DL(m) 2d → F
(m) 2d .
Here the branch divisor does not contain the (−2)-part. Theorem 4.2 below shows that there are only five exceptional varieties SF2(dm) with m > 0 and d >1 that are possibly not of general type.
The variety SF2(2)d can be interpreted as the moduli space of K3 surfaces of
degree 2d with spin structure: see [GHS2, §5]. The three-fold SF2(0)d is the moduli space of (1, t)-polarised abelian surfaces.
Theorem 4.2 The varietySF(2md)is of general type for anyd >1ifm≥3. If
m= 2thenSF2(2)d is of general type ifd≥3. Ifm= 1thenSF
(1)
2d is of general type if d= 5 ord≥7.
Proof. The case m = 2 is [GHS2, Theorem 5.1], and the result form ≥5 is immediate from Theorem 1.1. For m = 1, 3 and 4 we can prove more than what follows from Theorem 1.1.
The branch divisor ofSF(2md) is defined by the reflections in vectorsr ∈L
(m) 2d
such that −σr ∈ SOf
+
(L(2md)), because the rank of L(2md) is odd. Therefore r2=
−2d, by Proposition 2.1. If F ∈ M2k+1(SOf
+
(L(2md))) is a modular form (note that the character det is trivial),d >1 andz∈ D•
L(m)2d is such that (z, r) = 0, then
F(z) =F(−σr(z)) =F(−z) = (−1)2k+1F(z).
To apply the low-weight cusp form trick used in the proof of Theorem 1.1 one needs a cusp form of weight smaller than dimSF(2md) = 8m+ 3. By Proposition 3.1 there exists a cusp form F11+4m ∈ S11+4m(SOf
+
(L(2md))). For m ≥ 3 we have that 11 + 4m < 8m+ 3. Therefore the differential forms Fk
11+4mF(4m−8)k(dZ)k, for arbitrary F(4m−8)k ∈ M(4m−8)k(SOf
+
(L(2md))), ex-tend to the toroidal compactification of SF(2md) constructed in Theorem 1.3.
This proves the casesm≥3 of the theorem.
For the casem= 1 we use a cusp form of weight 9 with respect to SOf+(L(1)2d)
constructed in Proposition 3.1. ✷
We can obtain some information also for some of the remaining cases.
Proposition 4.3 The spacesSF8(1)andSF (1)
12 have non-negative Kodaira
di-mension.
Proof. By Proposition 3.1 there are cusp forms of weight 11 for SOf+(L(1)8 ) and SOf+(L(1)12). The weight of these forms is equal to the dimension. By the well-known observation of Freitag [F, Hilfssatz 2.1, Kap. III] these cusp forms determine canonical differential forms on the 11-dimensional varieties SF8(1)
andSF12(1). ✷
These varieties may perhaps have intermediate Kodaira dimension as it seems possible that a reflective modular form of canonical weight exists forL(1)8 and L(1)12.
In [GHS2] we used pull-backs of the Borcherds modular form Φ12onDII2,26 to
show that many moduli spaces of K3 surfaces are of general type. We can also use Borcherds products to prove results in the opposite direction.
Theorem 4.4 The Kodaira dimension ofFII(m) is−∞form= 0,1and2. Proof. For m = 0 we can see immediately that the quotient is rational: a straightforward calculation gives thatFII(0) = Γ\H1×H1whereH1 is the usual upper half plane and Γ is the degree 2 extension of SL(2,Z)×SL(2,Z) by the involution which interchanges the two factors. Compactifying this, we obtain the projective planeP2.
Form= 1, 2 we argue differently. There are modular forms similar to Φ12for the even unimodular lattices II2,10 and II2,18. They are Borcherds products Φ252and Φ127of weights 252 and 127 respectively, defined by the automorphic functions
∆(τ)−1(τ)E4(τ)2=q−1+ 504 +q(. . .) and
where q = exp(2πiτ) and ∆(τ) andE4(τ) are the Ramanujan delta function and the Eisenstein series of weight 4 (see [B1]). The divisors of Φ252 and Φ127 coincide with the branch divisors ofFII(1)andFII(2) defined by the (−2)-vectors. Moreover Φ252and Φ127each vanishes with order one along the respective divi-sor. Therefore ifF10k(dZ)k (orF18k(dZ)k) defines a pluricanonical differential
form on a smooth model of a toroidal compactification of FII(1) or F
(2)
II , then
F10k (or F18k) is divisible by Φk252 (or Φk127), since F10k or F18k must vanish
to order at leastkalong the branch divisor. This is not possible, because the quotient would be a holomorphic modular form of negative weight. ✷
We have already remarked that the spaceFII(2) is the moduli space of K3 sur-faces with an elliptic fibration with a section. Using the Weierstrass equations it is then clear that this moduli space is unirational (such K3 surfaces are parametrised by a linear system in the weighted projective spaceP(4,6,1,1)). In fact it is even rational: see [Le].
References
[BHPV] W. Barth, K. Hulek, C. Peters, A. Van de Ven,Compact complex sur-faces. Ergebnisse der Mathematik und ihrer Grenzgebiete4. Springer-Verlag, Berlin, 2004.
[B1] R.E. Borcherds,Automorphic forms on Os+2,2(R) and infinite
prod-ucts.Invent. Math.120(1995), 161–213.
[B2] R.E. Borcherds,Automorphic forms with singularities on Grassmani-ans.Invent. Math.132(1998), 491–562.
[BB] W.L. Baily Jr., A. Borel, Compactification of arithmetic quotient of bounded domains. Ann. Math84(1966), 442–528.
[CM] A. Clingher, J.W. Morgan, Mathematics underlying the F-theory/heterotic string duality in eight dimensions. Comm. Math. Phys.254(2005), 513–563.
[Dol] I.V. Dolgachev,Mirror symmetry for lattice polarized K3 surfaces. J. Math. Sci.81(1996), 2599–2630.
[E] M. Eichler, Quadratische Formen und orthogonale Gruppen. Die Grundlehren der mathematischen Wissenschaften 63. Springer-Verlag, Berlin 1952.
[EZ] M. Eichler, D. Zagier,The theory of Jacobi forms. Progress in Math-ematics55. Birkh¨auser, Boston 1985.
[F] E. Freitag, Siegelsche Modulfunktionen. Grundlehren der mathe-matischen Wissenschaften 254. Springer-Verlag, Berlin–G¨ottingen– Heidelberg, 1983.
[G2] V. Gritsenko,Modular forms and moduli spaces of abelian and K3 sur-faces.Algebra i Analiz6, 65–102; English translation in St. Petersburg Math. J.6 (1995), 1179–1208.
[GH] V. Gritsenko, K. Hulek, Minimal Siegel modular threefolds. Math. Proc. Cambridge Philos. Soc.123(1998), 461–485.
[GHS1] V. Gritsenko, K. Hulek, G.K. Sankaran, The Hirzebruch–Mumford volume for the orthogonal group and applications. Doc. Math. 12
(2007), 215–241.
[GHS2] V. Gritsenko, K. Hulek, G.K. Sankaran, The Kodaira dimension of the moduli of K3 surfaces. Invent. Math.169(2007), 519–567. [GHS3] V. Gritsenko, K. Hulek, G.K. Sankaran, Moduli spaces of
ir-reducible symplectic manifolds. MPI Preprint 2008-20 (2008),
arXiv:0802.2078.
[Ko] S. Kondo,Moduli spaces of K3 surfaces.Compositio Math.89(1993), 251–299.
[Le] P. Lejarraga,The moduli of Weierstrass fibrations over P1:
rational-ity. Rocky Mountain J. Math.23(1993), 649–650.
[Mum] D. Mumford, Hirzebruch’s proportionality principle in the non-compact case. Invent Math.42(1977), 239–277.
[Nik1] V.V. Nikulin, Finite automorphism groups of K¨ahler K3 surfaces.
Trudy Moskov. Mat. Obshch. 38(1979), 75–137. English translation in Trans. Mosc. Math. Soc.2, 71-135 (1980).
[Nik2] V.V. Nikulin, Integral symmetric bilinear forms and some of their applications. Izv. Akad. Nauk USSR43(1979), 105 - 167 (Russian); English translation in Math. USSR, Izvestiia14(1980), 103–166. [SZ] N.P. Skoruppa, D. ZagierJacobi forms and a certain space of modular
forms. Invent. Math.94(1988), 113–146.
V. A. Gritsenko Universit´e Lille 1
Laboratoire Paul Painlev´e
F-59655 Villeneuve d’Ascq, Cedex France
valery.gritsenko@math.univ-lille1.fr
K. Hulek
Institut f¨ur Algebraische Geometrie Leibniz Universit¨at Hannover D-30060 Hannover
Germany
hulek@math.uni-hannover.de
G. K. Sankaran
Department of Mathematical Sciences University of Bath
Bath BA2 7AY England
The Euler Characteristic of a Category
Tom Leinster1
Received: May 11, 2007 Revised: February 20, 2008
Communicated by Stefan Schwede
Abstract. The Euler characteristic of a finite category is defined and shown to be compatible with Euler characteristics of other types of object, including orbifolds. A formula is proved for the cardinality of a colimit of sets, generalizing the classical inclusion-exclusion formula. Both rest on a generalization of Rota’s M¨obius inversion from posets to categories.
2000 Mathematics Subject Classification: 18F99, 55U99, 05C50, 57N65.
Keywords and Phrases: Euler characteristic, finite category, inclusion-exclusion, M¨obius inversion, cardinality of colimit.
Introduction
We first learn of Euler characteristic as ‘vertices minus edges plus faces’, and later as an alternating sum of ranks of homology groups. But Euler character-istic is much more fundamental than these definitions make apparent, as has been made increasingly explicit over the last fifty years; it is something akin to cardinality or measure. More precisely, it is the fundamental dimensionless quantity associated with an object.
The very simplest context for Euler characteristic is that of finite sets, and of course the fundamental way to assign a quantity to a finite set is to count its elements. Euler characteristic of topological spaces can usefully be thought of as a generalization of cardinality; for instance, it obeys the same laws with respect to unions and products.
1Partially supported by a Nuffield Foundation award NUF-NAL 04 and an EPSRC
In a more sophisticated context, integral geometry, Euler characteris-tic also emerges clearly as the fundamental dimensionless invariant. A sub-set of Rn is polyconvex if it is a finite union of compact convex subsets.
Let Vn be the vector space of finitely additive measures, invariant under
Euclidean transformations, defined on the polyconvex subsets of Rn.
Had-wiger’s Theorem [KR] states that dimVn = n+ 1. (See also [Sc2], and [MS]
for an important application to materials science.) A natural basis con-sists of one d-dimensional measure for each d ∈ {0, . . . , n}: for instance,
{Euler characteristic,perimeter,area}whenn= 2. Thus, up to scalar multipli-cation, Euler characteristic is the unique dimensionless measure on polyconvex sets.
Schanuel [Sc1] showed that for a certain category of polyhedra, Euler char-acteristic is determined by a simple universal property, making its fundamental nature transparent.
All of the above makes clear the importance of defining and understanding Euler characteristic in new contexts. Here we do this for finite categories.
Categories are often viewed as large structures whose main purpose is organizational. However, some different viewpoints will be useful here. A combinatorial point of view is that a category is a directed graph (objects and arrows) equipped with some extra structure (composition and identities). We will concentrate on finite categories (those with only finitely many objects and arrows), which also suits the combinatorial viewpoint, and the composition and identities will play a surprisingly minor role.
A topological point of view is that a category can be understood through its classifying space. This is formed by starting with one 0-cell for each object, then gluing in one 1-cell for each arrow, one 2-cell for each commutative triangle, and so on.
Both of these points of view will be helpful in what follows. The topological perspective is heavily used in the sequel [BL] to this paper.
With topology in mind, one might imagine simply transporting the defini-tion of Euler characteristic from spaces to categories via the classifying space functor, as with other topological invariants: given a category A, define χ(A) as the Euler characteristic of the classifying space BA. The trouble with this is that the Euler characteristic of BAis not always defined. Below we give a definition of the Euler characteristic of a category that agrees with the topo-logical Euler characteristic when the latter exists, but is also valid in a range of situations when it does not. It is a rational number, not necessarily an integer. A version of the definition can be given very succinctly. LetAbe a finite category; totally order its objects as a1, . . . , an. Let Z be the matrix whose
(i, j)-entry is the number of arrows from ai to aj. Let M = Z−1, assuming
that Z is invertible. Thenχ(A) is the sum of the entries ofM. Of course, the reader remains to be convinced that this definition is the right one.
hom-set has at most one element: the objects are the elements of the pohom-set, and there is an arrow a ✲ b if and only if a ≤ b.) This leads, among other things, to a ‘representation formula’: given any functor known to be a sum of representables, the formula tells us the representation explicitly. This in turn can be used to solve enumeration problems, in the spirit of Rota’s paper.
However, the main application of this generalized M¨obius inversion is to the theory of the Euler characteristic of a category (§2). We actually use a dif-ferent definition than the one just given, equivalent to it whenZ is invertible, but valid for a wider class of categories. It depends on the idea of the ‘weight’ of an object of a category. The definition is justified in two ways: by showing that it enjoys the properties that the name would lead one to expect (behaviour with respect to products, fibrations, etc.), and by demonstrating its compati-bility with Euler characteristics of other types of structure (groupoids, graphs, topological spaces, orbifolds). There is an accompanying theory of Lefschetz number.
The technology of M¨obius inversion and weights also solves another prob-lem: what is the cardinality of a colimit? For example, the union of a family of sets and the quotient of a set by a free action of a group are both examples of colimits of set-valued functors, and there are simple formulas for their cardi-nalities. (In the first case it is the inclusion-exclusion formula.) We generalize, giving a formula valid for any shape of colimit (§3).
Rota and his school proved a large number of results on M¨obius inversion for posets. As we will see repeatedly, many are not truly order-theoretic: they are facts about categories in general. In particular, important theorems in Rota’s original work [R] generalize from posets to categories (§4).
(The body of work on M¨obius inversion in finite lattices is not, however, so ripe for generalization: a poset is a lattice just when the corresponding category has products, but a finite category cannot have products unless it is, in fact, a lattice.)
Other authors have considered different notions of M¨obius inversion for cat-egories; notably, there is that developed by Content, Lemay and Leroux [CLL] and independently by Haigh [H]. This generalizes both Rota’s notion for posets and Cartier and Foata’s for monoids [CF]. (Here a monoid is viewed as a one-object category.) The relation between their approach and ours is discussed in §4. Further approaches, not discussed here, were taken by D¨ur [D] and L¨uck [L¨u].
In the case of groupoids, our Euler characteristic of categories agrees with Baez and Dolan’s groupoid cardinality [BD]. The cardinality of the groupoid of finite sets and bijections is e = 2.718. . ., and there are connections to ex-ponential generating functions and the species of Joyal [J, BLL]. Par´e has a definition of the cardinality of an endofunctor of the category of finite sets [Pa]; I do not know whether this can be related to the definition here of the Lefschetz number of an endofunctor.
is nothing new: see for instance Wall [Wl], Bass [Ba] and Cohen [Co], and the discussion of orbifolds in§2.
Ultimately it would be desirable to have the Euler characteristic of cate-gories described by a universal property, as Schanuel did for polyhedra [Sc1]. For this, it may be necessary to relax the constraints of the present work, where for simplicity our categories are required to be finite and the coefficients are required to lie in the ring of rational numbers. Rather than asking, as below, ‘does this category have Euler characteristic (inQ)?’, we should perhaps ask ‘in what rig (semiring) does the Euler characteristic of this category lie?’ However, this is not pursued here.
Acknowledgements I thank John Baez, Andy Baker, Nick Gurski, Ieke Moerdijk, Urs Schreiber, Ivan Smith and Stephen Watson for inspiration and useful discussions. I am grateful to the Centre de Recerca Matem`atica, Barcelona, for their hospitality. I also thank the very helpful referee.
1 M¨obius inversion
We consider a finite categoryA, writing obAfor its set of objects and, whena andb are objects,A(a, b) for the set of maps fromatob.
Definition 1.1 We denote by R(A) the Q-algebra of functions obA ×
obA ✲ Q, with pointwise addition and scalar multiplication, multiplica-tion defined by
(θφ)(a, c) =X
b∈A
θ(a, b)φ(b, c)
(θ, φ∈R(A),a, c∈A), and the Kroneckerδas unit.
The zeta function ζA = ζ ∈ R(A) is defined by ζ(a, b) = |A(a, b)|. If ζ is invertible in R(A) then A is said to have M¨obius inversion; its inverse µA=µ=ζ−1 is theM¨obius function ofA.
If a total ordering is chosen on the n objects of A then R(A) can be regarded as the algebra of n×n matrices over Q. The defining equations of the M¨obius function are
X
b
µ(a, b)ζ(b, c) =δ(a, c) =X
b
ζ(a, b)µ(b, c)
for alla, c∈A. By finite-dimensionality,µζ=δ if and only ifζµ=δ.
The definitions above could be made for directed graphs rather than cat-egories, since they do not refer to composition. However, this generality seems to be inappropriate. For example, the definition of M¨obius inversion will lead to a definition of Euler characteristic, and if we use graphs rather than categories then we obtain something other than ‘vertices minus edges’. Proposition 2.10 clarifies this point.
Examples 1.2 a. Any finite posetAhas M¨obius inversion; this special case was investigated by Rota [R] and others. We may compute µ(a, c) by induction on the number of elements betweenaandc:
µ(a, c) =δ(a, c)− X
b:a≤b<c
µ(a, b).
In particular,µ(a, c) = 0 unlessa≤c, and µ(a, a) = 1 for alla. See also Theorem 1.4 and Corollary 1.5.
b. LetM be a finite monoid, regarded as a category with unique object⋆. (The arrows of the category are the elements of the monoid, and composi-tion in the category is multiplicacomposi-tion in the monoid.) Thenζ(⋆, ⋆) =|M|, soµ(⋆, ⋆) = 1/|M|.
c. LetN≥0. WriteDinj
N for the category with objects 0, . . . , N whose maps
a ✲ b are the order-preserving injections {1, . . . , a} ✲ {1, . . . , b}. Thenζ(a, b) = ab, and it is easily checked that µ(a, b) = (−1)b−a b
a
. If we use surjections instead of injections thenζ(a, b) = ab−−11andµ(a, b) = (−1)a−b a−1
b−1
.
A category with M¨obius inversion must be skeletal (isomorphic objects must be equal), for otherwise the matrix of ζ would have two identical rows. The property of having M¨obius inversion is not, therefore, invariant under equivalence of categories.
In general we cannot hope to just spot the M¨obius function of a category. In 1.3–1.7 we make tools for computing M¨obius functions. These cover large classes of categories, although not every finite skeletal category has M¨obius inversion (1.11(d), (e)).
Letn≥0, letA be a category or a directed graph, and leta, b∈A. An n-path fromatob is a diagram
a=a0
f1✲
a1
f2✲
· · · fn✲ an=b (1)
inA. It is acircuit ifa=b, and (whenAis a category)nondegenerate if nofi
is an identity.
Lemma 1.3 The following conditions on a finite categoryAare equivalent: a. every idempotent inAis an identity
b. every endomorphism inAis an automorphism c. every circuit inAconsists entirely of isomorphisms.
Proof (a) ⇒ (b) follows from the fact that if f is an element of a finite monoid then some positive power off is idempotent. The other implications
Theorem 1.4 LetAbe a finite skeletal category in which the only idempotents are identities. Then Ahas M¨obius inversion given by
µ(a, b) =X(−1)n/
|Aut(a0)| · · · |Aut(an)|
where Aut(a) is the automorphism group of a∈A and the sum runs over all
n≥0 and paths (1) for which a0, . . . , an are all distinct.
Proof First observe that for a path (1) inA, ifa06=a16=· · · 6=an then the
ais are all distinct. Indeed, if 0 ≤i < j ≤n and ai = aj then the sub-path
running from ai to aj is a circuit, so by Lemma 1.3, fi+1 is an isomorphism, and by skeletality,ai=ai+1.
Now leta, c∈Aand defineµby the formula above. We have
X
b∈A
µ(a, b)ζ(b, c) = µ(a, c)ζ(c, c) + X
b:b6=c
µ(a, b)ζ(b, c)
= |Aut(c)|
µ(a, c) +
X
b:b6=c, g∈A(b,c)
µ(a, b)/|Aut(c)|
= |Aut(c)|nµ(a, c) +X(−1)n/|Aut(a0)| · · · |Aut(an)||Aut(c)| o
,
where the last sum is over alln≥0 and paths
a=a0
f1✲
· · · fn✲ an=b g✲
c
such thata0 6=· · · 6=an 6=c. By definition ofµ, the term in braces collapses
to 0 if a6=c and to 1/|Aut(a)| ifa=c. Hence Pbµ(a, b)ζ(b, c) =δ(a, c), as
required.
Corollary 1.5 LetAbe a finite skeletal category in which the only endomor-phisms are identities. ThenAhas M¨obius inversion given by
µ(a, b) =X
n≥0
(−1)n|{nondegenerate n-paths fromatob}| ∈Z.
WhenAis a poset, this is Philip Hall’s theorem (Proposition 3.8.5 of [St] and Proposition 6 of [R]).
An epi-mono factorization system (E,M) on a category A consists of a classE of epimorphisms inAand a classMof monomorphisms inA, satisfying axioms [FK]. The axioms imply that every mapf inAcan be expressed asme for some e∈ E and m∈ M, and that this factorization is essentially unique: the other pairs (e′, m′) ∈ E × M satisfying m′e′ = f are those of the form
Theorem 1.6 LetAbe a finite skeletal category with an epi-mono factorization system(E,M). Then Ahas M¨obius inversion given by
µ(a, b) =X(−1)n/|Aut(a0)| · · · |Aut(an)|
where the sum is over all n ≥ r ≥ 0 and paths (1) such that a0, . . . , ar are distinct,ar, . . . , an are distinct, f1, . . . , fr∈ M, andfr+1, . . . , fn∈ E.
Proof The objects ofAand the arrows inE determine a subcategory of A, also denoted E; it satisfies the hypotheses of Theorem 1.4 and therefore has M¨obius inversion. The same is true ofM.
Any element α∈QobA =Q
a∈AQgives rise to an element of R(A), also denotedαand defined by α(a, b) =δ(a, b)α(b). This defines a multiplication-preserving map fromQobAtoR(A), where the multiplication onQobAis coor-dinatewise. We have elements |Aut|and 1/|Aut|ofQobA, where, for instance,
|Aut|(a) =|Aut(a)|.
By the essentially unique factorization property,ζA=ζE·|Aut1 |·ζM. Hence
Ahas M¨obius functionµA=µM· |Aut| ·µE. Theorem 1.4 applied to µMand
µE then gives the formula claimed.
Example 1.7 LetN ≥ 0 and writeFN for the full subcategory ofSet with
objects 1, . . . , N, where ndenotes a (chosen) n-element set. Let E be the set of surjections in FN and Mthe set of injections; then (E,M) is an epi-mono
factorization system. Theorem 1.6 gives a formula for the inverse of the matrix (ij)
i,j. For instance, take N = 3; thenµ(1,2) may be computed as follows:
Paths Contribution to sum
1✲ 2✲ 2 −2/1!2! =−1
1✲ 3✲ 3 ✲6✲ 2 3·6/1!3!2! = 3/2 1✲ 2✲ 2✲6✲ 3 ✲6✲ 2 −2·6·6/1!2!3!2! =−3
Here ‘1✲2✲ 2’ means that there are 2 monomorphisms from 1 to 2, ‘3 ✲6✲ 2’ that there are 6 epimorphisms from 3 to 2, etc. Henceµ(1,2) =−1 + 3/2−3 =
−5/2.
One of the uses of the M¨obius function is to calculate Euler character-istic (§2). Another is to calculate representations. Specifically, suppose that we have a Set-valued functor known to be familially representable, that is, a coproduct of representables. The Yoneda Lemma tells us that the family of representing objects is unique (up to isomorphism). But if we have M¨obius inversion, there is actually a formula for it:
Proposition 1.8 Let A be a finite category with M¨obius inversion and let
X :A ✲ Setbe a functor satisfying
X ∼=X
a
for some natural numbersr(a)(a∈A). Then
r(a) =X
b
|X(b)|µ(b, a)
for all a∈A.
In the first formula,Pdenotes coproduct ofSet-valued functors.
Proof Follows from the definition of M¨obius function.
In the spirit of Rota’s programme, this can be applied to solve counting problems, as illustrated by the following standard example.
Example 1.9 Aderangement is a permutation without fixed points. We cal-culatedn, the number of derangements ofnletters.
Fix N ≥ 0. Take the category DinjN of Example 1.2(c) and the functor S:DinjN ✲ Setdefined as follows: S(n) isSn, the underlying set of thenth
symmetric group, and iff ∈Dinj
N(m, n) andτ ∈Sm, the induced permutation
Sf(τ)∈Sn acts asτ on the image of f and fixes all other points. Any
permu-tation consists of a derangement together with some fixed points, so there is an isomorphism of sets
Sn∼= X
m
dmDinjN(m, n)
wherePdenotes disjoint union. Then by Proposition 1.8 and Example 1.2(c),
dn= X
m
|Sm|µ(m, n) = X
m
m!(−1)n−m
n
m
=n!
1
0!− 1
1!+· · ·+ (−1)n
n!
.
To set up the theory of Euler characteristic we will not need the full strength of M¨obius invertibility; the following suffices.
Definition 1.10 LetA be a finite category. Aweighting on Ais a function k• : obA ✲ Qsuch that for alla
∈A,
X
b
ζ(a, b)kb= 1.
Acoweighting k• onAis a weighting onAop.
Note thatAhas M¨obius inversion if and only if it has a unique weighting, if and only if it has a unique coweighting; they are given by
ka=X
b
µ(a, b), kb= X
a
Examples 1.11 a. LetLbe the category
b. LetM be a finite monoid, regarded as a category with unique object⋆. Again there is a unique weightingk•, withk⋆= 1/
|M|.
c. If Ahas a terminal object 1 thenδ(−,1) is a weighting onA.
d. A finite category may admit no weighting at all. (This can happen even when the category is Cauchy-complete, in the sense defined in the Ap-pendix.) An example is the categoryAwith objects and arrows
a1 a2
ak and neither p nor q is an identity then
q◦p=fik.
e. A category may certainly have more than one weighting: for instance, ifA is the category consisting of two objects and a single isomorphism between them, a weighting onAis any pair of rational numbers whose sum is 1. But even a skeletal category may admit more than one weighting. Indeed, the full subcategories B ={a1, a2} and C ={a1, a2, a3} of the categoryA of the previous example both have a 1-dimensional space of weightings.
In contrast to M¨obius invertibility, the property of admitting at least one weighting is invariant under equivalence:
Proof Let F : A ✲ B be an equivalence. Given a∈A, write Ca for the
number of objects in the isomorphism class ofa. Take a weightingl•onBand
put ka= (P
b:b∼=F(a)lb)/Ca. I claim thatk• is a weighting onA.
To prove this, choose representativesa1, . . . , amof the isomorphism classes
of objects of A; then F(a1), . . . , F(am) are representatives of the isomorphism
classes of objects ofB. Let a′ ∈A. For anya∈A, the numbersζ(a′, a) andka
depend only on the isomorphism class ofa. Hence
X
a∈A
ζ(a′, a)ka =
m X
i=1
X
a:a∼=ai
ζ(a′, a)ka
=
m X
i=1
Caiζ(a′, ai)kai
=
m X
i=1
X
b:b∼=F(ai)
ζ(a′, ai)lb
= X
b∈B
ζ(F(a′), b)lb
= 1,
as required.
Weightings and M¨obius functions are compatible with sums and products of categories. We write Pi∈IAi for the sum of a family (Ai)i∈I of categories,
also called the coproduct or disjoint union and written`i∈IAi. The following
lemma is easily verified.
Lemma 1.13 Let n≥0and letA1, . . . ,An be finite categories. a. If each Ai has a weighting k•i then
P
iAi has a weighting l• given by
la =ka
i whenever a∈Ai. If each Ai has M¨obius inversion then so does P
iAi, where fora∈Ai andb∈Aj,
µPAk(a, b) =
µAi(a, b) if i=j
0 otherwise.
b. If each Ai has a weighting k•i then QiAi has a weighting l• given by
l(a1,...,an) = ka1
1 · · ·kann . If each Ai has M¨obius inversion then so does Q
iAi, with
µQAi((a1, . . . , an),(b1, . . . , bn)) =µA1(a1, b1)· · ·µAn(an, bn).
To every Set-valued functor X there is assigned a ‘category of elements’
E(X). (See the Appendix for a review of definitions.) This is also true of functorsX taking values inCat, the category of small categories and functors, even when X is only a weak or ‘pseudo’ functor. We say that aSet- orCat -valued functor X is finite ifE(X) is finite. When the domain category Ais finite, this just means that each set or categoryX(a) is finite.
Lemma 1.14 Let A be a finite category and X : A ✲ Cat a finite weak functor. Suppose that we have weightings on A and on eachX(a), all written
k•. Then there is a weighting on E(X) defined by k(a,x) = kakx (a ∈ A,
x∈X(a)).
Proof Leta∈Aandx∈X(a). Then
X
(b,y)∈E(X)
ζ((a, x),(b, y))kbky = X
b X
f∈A(a,b)
X
y∈X(b)
ζ((X(f))x, y)ky
kb
= X
b
ζ(a, b)kb= 1.
This result will be used to show how Euler characteristic behaves with respect to fibrations.
2 Euler characteristic
In this section, the Euler characteristic of a category is defined and its basic properties are established. The definition is justified by a series of propositions showing its compatibility with the Euler characteristics of other types of object: graphs, topological spaces, and orbifolds. There follows a brief discussion of the Lefschetz number of an endofunctor.
Lemma 2.1 LetAbe a finite category,k• a weighting onA, andk
•a
coweight-ing on A. ThenPaka=P aka. Proof
X
b
kb=X
b X
a
kaζ(a, b) !
kb=X
a
ka X
b
ζ(a, b)kb
!
=X
a
ka.
If A admits a weighting but no coweighting thenPaka may depend on