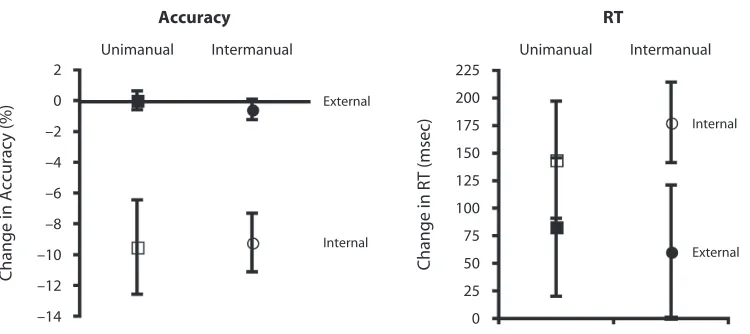
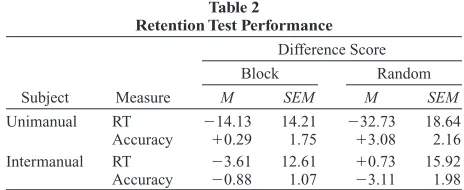
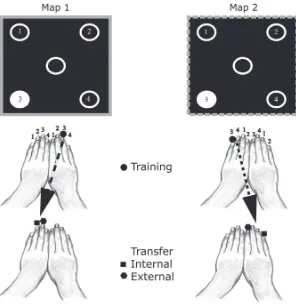
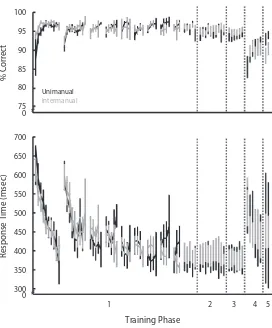
On the minimization of task switch costs (1)
Teks penuh
Gambar

Garis besar
Dokumen terkait
Melakukan promosi seperti membuat kalender yang bergambarkan produk Donaldson, membagikan katalog- katalog bergambarkan produk-produk ciri khas dari Donalson ke
The indicators of mathematically creative thinking in this study were (1) fluency characteristics that elicit many ideas, answers, solution of the problems, or
MEMS sensors of the SMAMID FUs have a digital output expressed in bits and each output is converted to quantities measured in terms of the gravity acceleration g by means of a
[r]
[r]
Unit Layanan Pengadaan Bar ang/ Jasa pada Lembaga Pener bangan dan Antar iksa Nasional (LAPAN) akan melaksanakan Pelelangan Seder hana dengan pascakualifikasi untuk
Kepatuhan wajib pajak di KPP Pratama wilayah Kota Bandung dan Cimahi belum maksimal sesuai harapan Direktorat Jendral Pajak, hal itu ditandai dengan masih adanya
Pada hari ini Rabu tanggal tujuh bulan Oktober tahun Dua ribu lima belas , pada jam 8 00 wib Pokja ULP II PSTW “ Budhi Dharma ” Bekasi telah melakukan evaluasi dokumen