Bab 9 Transformasi Laplace

KK-Astronomi ITB Page 9-1

Bab 9

Transformasi Laplace

____________________________________________________________________

9-1. Definisi Transformasi Laplace

Misalkan f(t) suatu fungsi real dengan variable t dan t>0. Transformasi Laplace didefinisikan sebagai:





0 0 ( ) ( ) lim ( ) ( ) , 0 T st st T L f t F s f t e dt f t e dt           









s=j, j= 1,  dan  variable real

definisi: jika f(t) didefinisikan dan berharga tunggal untuk t>0 dan F(s) konvergen mutlak. Untuk bilangan real 0, maka f(t) dikatakan dapat ditransformasikan secara

Laplace (Laplace-transformable), bila

0 _lim 0 0 0 ( ) ( ) T t t T f t e  dt f t e  dt         





 , 0  T

Contoh: f t( )etadalah Laplace Transformable sebab,

0 0 ( 0 1) 0 0 0 ( ) t t t t f t e  dt e e  dt e  dt       _   _  







0 ( 1) 0 0 0 1 1 (1 ) 1 t e             

9-2. Definisi Transformasi Laplace inversi

Misalkan F(s) transformasi Laplace dari fungsi f(t), t>0. Maka transformasi Laplace invers adalah





1 0 1 ( ) ( ) ( ) , 1, 2 C j st C j F s f t F s e ds j c j         



   L

KK-Astronomi ITB Page 9-2

9-3 Sifat-sifat transformasi Laplace (TL)

1. Sifat linearitas ( ai suatu konstanta real)



 

 











1 1 2 2 1 1 2 2 1 1 2 2 1 1 2 2 a f (t) + a f (t) = a f (t) a f (t) a f (t) a f (t) a F s( ) a F s( )      L L L L L

2. Transformasi Laplace dari turunan fungsi, df

dt ( ) (0 ) df sF s f dt   _{  }     L

3. TL dari fungsi integral

0

( )

t

f z dz



, dimana transformasi Laplace f(t) adalah F(s)

0 ( ) [ ( ) ] t F s f z dz s 



L 4. TL dari fungsi f(t )

a (time scaling) adalah L[ (f ta)]aF as( ),dimana ( ) [ ( )]

F s L f t

5. TL dari fungsi f(t-T) (time delay), T > 0 dan f(t-T)= 0 untuk tT [ (f t T )]esTF s( ),

L dimana F s( )L[ ( )]f t

6. TL dari fungsi eatf t( ),(komplek translation) L[eatf t( )]F s a(  ), dimana ( ) [ ( )]

F s L f t

7. TL hasil kali dua fungsi f t₁( ) dan f t₂( )

1 2 1 2 1 [ ( ) ( )] ( ) ( ) 2 f t f t F F s d j       

_



L (complex convolution integral)

9-4 Sifat-Sifat Transformasi Laplace invers (TLI)

1. TLI dari fungsi F(s )

a (frequency scalling) 1 [ (F s ] af at( ) a  _ L , dimana L1[ ( )]F s  f t( )

KK-Astronomi ITB Page 9-3 1 1 2 1 2 2 1 0 0 [ ( ). ( )] ( ) ( ) ( ) ( ) t t F s F s f z f t z dz f z f t z dz  _{   }





L dimana 1 1 1 1 2 2 [ ( )]F s f t( ), [F s( )] f t( )  _  _ L L dimana F s( )L[ ( )]f t

(complex convolution integral)

9-5 Ilustrasi

1. Carilah TL dari fungsi f t( )ete2t

Penyelesaian : 2 2 2 [ ] [ ] [ ] 0 0 1 1 2 3 2 1 2 _{3 2} t t t t t st t st e e e e e e dt e e dt s s s _{s s}    _  _  _  _{ }   _{ }         _{ } L L L

2. Carilah Transformasi Laplace Inversi dari fungsi ( ) 1 1 F s s   Penyelesaian : 1 1 1 1 1 2 1 st e ds s  j s     _{ }  _  _  



L Misal 1 x s dx ds s x          dans    x Jadi ( 1) 1 1 1 1 2 2 x t t xt e e e dx dx s  j x  j x         _{  }  _   





L

Pernyataan ini sukar untuk diselesaikan, tapi dengan mengingat bahwa L1



F s( )



 f t( ) dari contoh 1, dapat dikatakan ;

KK-Astronomi ITB Page 9-4 1 1 1 t e s      _   

L atau kita peroleh suatu pernyataan, Karena

1 1 2 1 1 1 2 2 2 t xt xt xt t e e t e e e dx e dx dxt s j x  j x  j xt          _{     }  _   







L

Karena bentuk xt dan x tidak akan mengubah batas integrasi bila x menuju ±∞ maka

2 1 2 x t e dx e j x    



Sulit dihitung secara langsung, tapi mudah dengan Transformasi Laplace inversi.

3. Carilah transformasi Laplace dari fungsi;

1 ( ) ( ) ( ) at bt f t e e b a      Jawab





1 ( ) ( ) ( ) 1 ( ) ( ) ( ) ( ) at bt at bt f t e e b a f t e e b a           _  _  L L L

Kita cari satu persatu dari komponen tersebut





( ) ( ) 0 0 0 ( ) 0 1 ( ) 1 1 1 0 1 ( ) ( ) ( ) at at st a s t a s t a s t e e e dt e dt de a s e a s a s a s                     _{ }  _{ }   _{ }      







L

Dengan cara yang sama, diperoleh (tinggal mengganti a dengan b)

1 bt e s b       _ L Maka

KK-Astronomi ITB Page 9-5



( )



1 ( ) ( ) ( ) at bt f t e e b a      _  _  L L L 1 1 1 1 ( ) ( )( ) s b s a b a s a s b b a s a s b         _  _ _{ }           1 . ( )( ) 1 ( )( ) b a b a s a s b s a s b        

4. Carilah transformasi Laplace dari fungsi;

1 ( ) ( ) ( ) ( ) at bt f t z a e z b e b a      _    _  Jawab;





1 ( ) ( ) ( ) ( ) 1 ( ) ( ) ( ) ( ) at bt at bt f t z a e z b e b a f t z a e z b e b a        _    _        _{ }  _ _  _  L L L





1 ( ) ( ) ( ) ( ) at bt f t z a e z b e b a         _  _{ }  _{ }  L L L





1 1 1 ( ) ( ) ( ) ( ) f t z a z b b a s a s b    _    _      L





1

_

1

_

(( )( )) (( )( )) ( ) ( ) ( )( ) z a z b s b z a s a z b f t b a s a s b b a s a s b             _  _ _{ }           L

KK-Astronomi ITB Page 9-6

















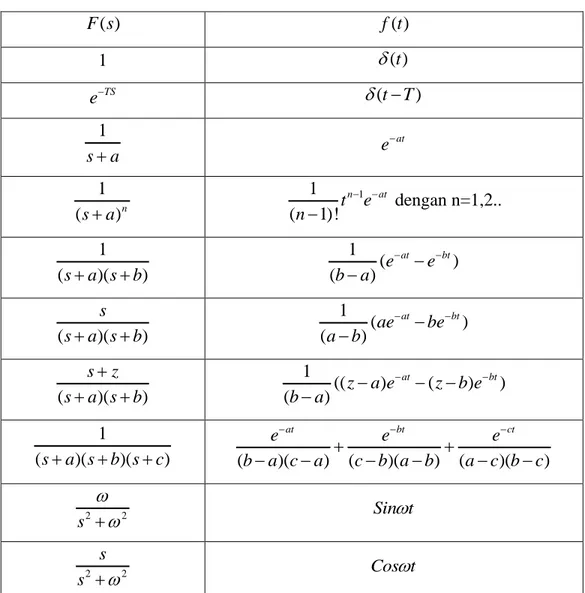
1 ( ) ( ) ( )( ) 1 ( )( ) 1 ( ) ( ) 1 ( )( ) ( )( ) ( )( ) ( )( ) sz as bz ab sz bs az ab b a s a s b bs as bz az b a s a s b s b a z b a b a s z b a s a s b b a s a s b s z s a s b           _{ }  _   _       _{ }  _   _           _{ } _{ }  _   _  _   _    

Dalam contoh yang diberikan ini dapat dibuat tabel transformasi Laplace untuk berbagai fungsi. Tabel ini diperlukan ketika kita mencari Transformasi Laplace inversi.

KK-Astronomi ITB Page 9-7

Tabel 9. 1 Transformasi Laplace( t>0)

( ) F s f t( ) 1 ( )t TS e (t T ) 1 sa at e 1 (sa)n 1 1 ( 1)! n at t e n    dengan n=1,2.. 1 (sa s b)(  ) 1 ( ) ( ) at bt e e b a  _   ( )( ) s sa s b 1 ( ) ( ) at bt ae be a b  _   ( )( ) s z s a s b    1 (( ) ( ) ) ( ) at bt z a e z b e b a       1 (sa s b s c)(  )(  ) ( )( ) ( )( ) ( )( ) at bt ct e e e b a c a c b a b a c b c            2 2 s    Sin t 2 2 s s  Cos t Perhatikan

 

 

 

 

 

 

 

 

0 0 0 ' ' _{( )} 0 0 0 st st st st f e f t dt e df t _{e f t} s e f t dt f s f s f f             _  _      







L L L

KK-Astronomi ITB Page 9-8

 

 

 

 



 

 



 

 

 

 

 

 

 

 



 

 

 



 

 

 

 

 

2 2 3 2 '' ' ' ' 0 0 ' 0 0 ' 0 ''' '' '' '' 0 0 ' 0 '' 0 0 ' 0 '' 0 d f f s f f s s f f f s f sf f dt d f f s f f s s f sf f f dt s f s f sf f    _{ }             _{ }            L L L L L L L L L L

Dengan induksi kita mempunyai bentuk umum;

 

 

 1

_{ }

 2

_{ }

_{ }

( ) 1 2 3 ( 1) 0 0 0 . . . 0 m m m m m m f s f s  f s  f s  f f            L L

9.6 Mencari solusi dengan bantuan table

Contoh (1) Tentukan TL dari fungsi f t

 

t2

Jawab : f

 

0 0, f ' 0

 

0, f '' 0

 

2 dan

 

2 2 s  L Jadi L

 

f '' s2L

 

f sf

 

0  f ' 0

 

2 s2

 

f t2 2₃ s  L L   s

Contoh (2) Tentukan TL dari fungsi f t

 

cos

 

t dan g t

 

sin

 

t

Jawab a) f t

 

cos

   

t ,f ' t  sin

 

t ,f ''

 

t  2cos

 

t  2f t

 

Jadi L

 

f '' s2L

 

f sf

 

0  f ' 0

 

 

 

2 2 f s f s   L  L 

 

f ₂s ₂ s    L

b) g t

 

sin

 

t ,g t'

 

cos

   

t , ''g t  2sin

 

t  2g t

 

L

 

g'' s2L

 

g sg

 

0 g' 0

 

2L[ ]g s2L

 

g  0 

 

g _{2 2} s     L

KK-Astronomi ITB Page 9-9

Contoh (3) : carilah TL dari fungsi 2

( ) sin

f t  t

Jawab:





2 '

( ) sin , ( ) 2sin cos sin 2

f t  t f t  t t  t Jadi L[f ] sL[ ]f  f(0)sL[ ]f atau ' 2 2 [ ] [sin 2 ] 2 [ ] ( 2 ) f t f s s s s     L L L

Contoh (4) : carilah TL dari fungsi f t( )tsint

Jawab: '

( ) sin , ( ) sin cos

f t t t f t   t t t " 2 " 2 ( ) 2 cos sin ( ) 2 cos ( ) f t t t t f t t f t            ' (0) 0, (0) 0 f  f  Sehingga L[f"]s2L[ ]f sf(0) f'(0)s2L[ ]f 2 2 2 2 2 2 [2 cos ( )] [ ] 2 [cos ] [ ] [ ] ( ) [ ] 2 [cos ] t f t s f t f s f s f t                 L L L L L L L Jadi



 

_{ }

_

2 2 2 2 2 2 2 _{2 2} 2 2 2 [ ]f [cos t] s s s s s _s  _      _       _ L L

Contoh (5) : Solusi persamaan diferensial dengan syarat awal

" ' 4 3 0 y  y  y , dengan y(0)3, (0) 1y'  Jawab: L[ ]y" L[4 ']y L[3 ]y 0



2







[ ] (0) '(0) 4 [ ] '(0) 3 [ ] 0 s L y sy y  sL y y  L y 



2







( ) 3 1 4 ( ) 3 3 ( ) 0 s Y s   s sY s   Y s  2 ( ) 4 ( ) 3 ( ) 3 1 12 3 13 s Y s  sY s  Y s  s   s



s3



s1



Y s( )3s13



3



13



2 5 ( ) 3 1 3 1 s Y s s s s s         

KK-Astronomi ITB Page 9-10 1 1 2 1 5 [ ( )] 3 1 Y s s s  _  _ _     _   _      L L L

 

1 1 1 1 3 2 5 2 5 3 1 t t y t e e s s           _{ } _{ }         L L Jadi solusinya 3 ( ) 2 t 5 t y t   e  e

9-6 Soal Latihan

Carilah solusi persamaan differensial homogen berikut dengan Transformasi Laplace. Dengan syarat y(0) = 1 dan y(0) = 0

(1) 2 2 cos x d y xe x dx   (2) 2 2 2 0 d y dy x x dx  dx  (3) 2 2 0 d y dy x x dx dx  (4) ₂ 2 0 2   y dx y d (5) 2 2 3 4 0 d y dy y dx  dx   (6) 2 2 3 0 d y dy dx  dx  (7) 2 2 4 13 0 d y dy y dx  dx 

KK-Astronomi ITB Page 9-11

Bab 9 ... 1

Transformasi Laplace... 1

9-1. Definisi Transformasi Laplace ... 1

9-2. Definisi Transformasi Laplace inversi ... 1

9-3 Sifat-sifat transformasi Laplace (TL) ... 2

9-4 Sifat-Sifat Transformasi Laplace invers (TLI) ... 2

9-5 Ilustrasi ... 3

9.6 Mencari solusi dengan bantuan table ... 8

Contoh (1) ... 8 Contoh (2) ... 8 Contoh (3) ... 9 Contoh (4) ... 9 Contoh (5) ... 9 9-6 Soal Latihan ... 10