PELAJARI TENTANG FUNGSI TRANSENDEN

8. FUNGSI

TRANSENDEN

8.1 Invers Fungsi

Misalkan dengan^{f : Df → Rf}

) ( x f y

x ! =

v

u ≠

^{f (u) ≠ f (v)}

Definisi 8.1 Fungsi y = f(x) disebut satu-satu jika f(u) = f(v) maka u = v atau jika maka

x y =

x y = −

x2

y =

u v

Secara geometri grafik fungsi satu-satu dan garis yang sejajar dengan sumbu x berpotongan di satu titik.

Teorema : Jika fungsi f satu-satu maka f mempunyai invers notasi f ⁻¹

f

f

D

R

f

⁻¹

: →

( ) ^y

f x

y ! =

⁻¹

Berlaku hubungan

x x

f

f

⁻¹

( ( )) =

y y

f

f (

⁻¹

( )) =

f f

f

R

f

R D

D

⁻¹

= ,

⁻¹

=

Df Rf

f

x y=f(x)

R R

−1

) f

1(y f

x = ⁻

Teorema : jika f monoton murni(selalu naik/selalu turun) maka f mempunyai invers

x x

f ( ) =

x x

f ( ) = −

) 2

(x x

f =

u v

f(x)=x

R x

x

f '( ) =1> 0,∀ ∈ f selalu naik

f(x)=-x

R x

x

f '( ) = −1< 0,∀ ∈ f selalu turun

⎩⎨

⎧

<

<

>

= >

= 0, 0

0 ,

2 0 ) (

' x

x x x

f

f naik untuk x>0 turun untuk x <0

−1

f

−1

f

−1

f ada ada tidak ada

Contoh : Diketahui f x x ( ) = x −

+ 1

a.  Periksa apakah f mempunyai invers 2 b. Jika ada, tentukan inversnya

Jawab

a. ( 2)2

) 1 .(

1 ) 2 .(

) 1 (

' +

−

−

= +

x

x x x

f x Df

x > ∀ ∈

= + 0,

) 2 (

3

2

Karena f selalu naik(monoton murni) maka f mempunyai invers b. Misal

2 1 +

= − x y x

1 2 = − + y x

xy 1

1 1 2

2 −

−

= −

⇒

−

−

=

− y

x y y

x y

x

1 1 ) 2

1 ( 1 ) 2

(

¹

1

−

−

= −

− ⇒

−

= −

⁻

−

x x x

y f y y

f

Suatu fungsi yang tidak mempunyai invers pada daerah asalnya dapat dibuat mempunyai invers dengan cara membatasi daerah asalnya.

) 2

(x x

f =

u v

−1

f tidak ada

R x ∈

Untuk

) 2

(x x

f =

Untuk x>0 ada f ⁻¹

) 2

(x x

f =

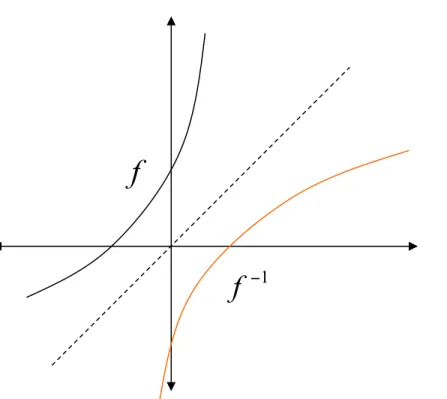
Grafik fungsi invers

Titik (x,y) terletak

pada grafik f Titik (y,x) terletak pada grafik f ⁻¹

Titik (x,y) dan (y,x) simetri terhadap garis y=x Grafik f dan semetri terhadap garis y=x f ⁻¹ f

−1

f

Turunan fungsi invers

Teorema Misalkan fungsi f monoton murni dan mempunyai turunan

pada selang I. Jika maka dapat diturunkan di y=f(x) dan

f ' ( x ) ≠ 0 , x ∈ I

_f ⁻¹

) ( ' ) 1

( )'

(

¹

x y f

f

⁻

=

Bentuk diatas dapat juga dituliskan sebagai

dx dy

dy dx

/

= 1

Contoh Diketahui tentukan f (x) = x⁵ + 2x +1 ( f ⁻¹)'(4)

2 5

) (

' x = x

⁴

+

f

,y=4 jika hanya jika x=1

1 1

Jawab :

Soal Latihan

f x x

x x

( ) = + 1 , >

0 f x( ) = ³ 2x −1

f x( ) = ⁵ 4x + 2

f x( ) = x , x

+ ≥

5

1 0

2

f x x ( ) = x +

− 1 1 3 ) 2

( −

= x x

f

Tentukan fungsi invers ( bila ada ) dari 1.

2.

3.

4.

5.

6.

8.2 Fungsi Logaritma Asli

n  Fungsi Logaritma asli ( ln ) didefinisikan sebagai :

n  Dengan Teorema Dasar Kalkulus II, diperoleh :

n  Secara umum, jika u = u(x) maka

ln x ,

t dt x

x

= ∫ ¹ > ⁰

1

[ ]

dt x D t

x D

x x x

1 ln 1

1

⎟ =

⎟

⎠

⎞

⎜ ⎜

⎝

= ⎛ ∫

[ ^u ] ^{D dt du}

D

x

u

1 1

ln

) (

⎟ =

⎜ ⎞

= ⎛ ∫

.

Contoh : Diberikan

^maka

Jika

Jadi,

Dari sini diperoleh :

Sifat-sifat Ln :

1. ln 1 = 0

2. ln(ab) = ln a + ln b 3. ln(a/b)=ln(a) – ln(b)

)) 2 4

ln(sin(

)

( x = x +

f

)) 2 4

(sin(

) 2 4

sin(

) 1 (

' +

= + D x

x x

f

_x

= 4 cot( 4 x + 2 )

0 ,|

|

ln ≠

= x x y

⎩⎨

⎧

<

−

= >

0 ,

) ln(

0 ,

ln

x x

x

x y x y 1x

'

ln → =

=

x y x

x

y 1 1

' )

ln( =

−

= −

→

−

=

. 0 1 ,

|)

|

(ln = x ≠

x x dx

d

∫ _x ^{1 dx = ln | x | + C}

a r

a

^r

ln ln

.

4 =

x dx

∫ x ₊

4

0

3 2

2

dx x

du x

u =

³

+ 2 → = 3

²

Contoh: Hitung jawab

Misal

2 2

3 2

3

2 x

du u

dx x x

∫ x ₊ ⁼ ∫ ⁼ ₃ ¹ ∫ _u ^{1 du = 1} ₃ ^{ln | u | + c}

c x + +

= ln | 2 | 3

1

₃

] ₀ ⁴

| 2

| 3 ln 1 2

3 4

0 3

2

+ + =

∫ _x ^{x dx x =} ₃ ^{1 (ln 66 − ln 2 ) = 1} ₃ ^{ln 33 .}

sehingga

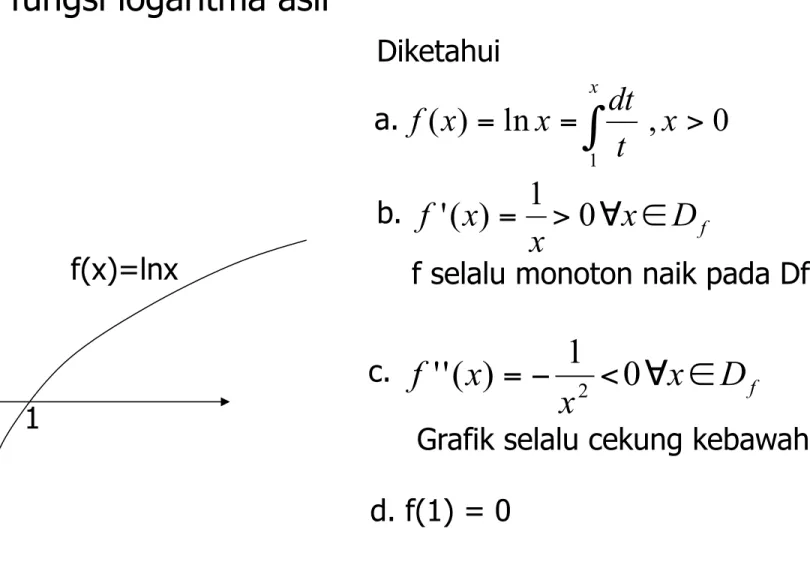
Grafik fungsi logaritma asli

0 ,

ln )

(

1

>

=

= ^x ∫ ^dt _t ^x

x f

x

D

f

x x x

f = 1 > 0 ∀ ∈ )

( '

f selalu monoton naik pada Df

D

f

x x x

f = − 1 < 0 ∀ ∈ )

(

''

₂

Diketahui a.

b.

c.

Grafik selalu cekung kebawah d. f(1) = 0

1

f(x)=lnx

8.3 Fungsi Eksponen Asli

n 

Karena maka fungsi logaritma asli monoton murni, sehingga mempunyai invers. Invers dari fungsi

logaritma asli disebut fungsi eksponen asli, notasi exp. Jadi berlaku hubungan

n 

Dari sini didapat : y = exp(ln y) dan x =ln(exp(x))

n 

Definisi 8.2 Bilangan e adalah bilangan Real positif yang bersifat ln e = 1.

Dari sifat (iv) fungsi logaritma diperoleh

[ ] ^{ln = 1 > 0 untuk x > 0 ,}

x x D

_x

y x

x

y = exp( ) ⇔ = ln

r e

r e

e

^r

= exp(ln

^r

) = exp ln = exp exp ( ^x ) = ^e

^x

e

x

dy y dx

dx

dy = = =

/ 1

x x

x

e e

D ( ) = ,

Jadi

y x

e

y =

^x

⇔ = ln

Turunan dan integral fungsi eksponen asli Dengan menggunakan turunan fungsi invers Dari hubungan

y dy

dx 1

=

' . )

( e

^{( )}

e u

D

_x ^{u x}

=

^u

Secara umum Sehingga

∫ ^e

^x

^{dx = e}

^x

^{+ C}

1

y=ln x y=exp (x)

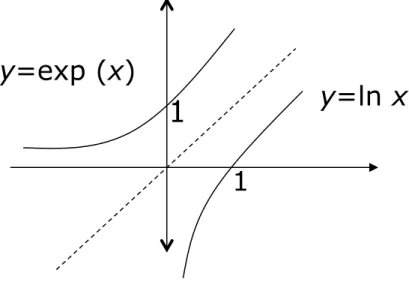
Grafik fungsi eksponen asli

Karena fungsi ekponen asli merupakan invers dari fungsi logaritma asli maka grafik fungsi eksponen asli diperoleh dengan cara mencerminkan grafik fungsi logaritma asli terhadap garis y=x

1

Contoh

) ln 3

( .

)

( e

^{3 ln}

e

^{3 ln}

D x x

D

^{x x}

=

^{x x}

= e

^3xlnx

( 3 ln x + 3 ).

3 . 1 3

1 3

1

_3/

2 / 3

c e

c e

du e x dx

e

^x _{u u x}

+

−

= +

−

=

−

= ∫

∫

Contoh Hitung

x dx e

^x

∫

²

/ 3

Jawab :

du x dx

x dx x du

u 3

1 1

3 3

2

2 → = −

= −

→ Misalkan =

Sehingga

)

))

(

( ( )

( x g x

^{h x}

f =

Penggunaan fungsi logaritma dan eksponen asli

Menghitung turunan fungsi berpangkat fungsi Diketahui

)) (

ln(

) ( ))

(

ln( f x = h x g x

))) (

ln(

) ( ( )))

(

(ln( f x D h x g x

D

_x

=

_x

) ( ) '

( ) )) (

( ln(

) ( ) '

( ) (

' g x

x g

x x h

g x

x h f

x

f = +

) ( )

( ) ' (

) )) (

( ln(

) ( ' )

(

' g x f x

x g

x x h

g x

h x

f ⎟⎟

⎠

⎞

⎜⎜⎝

⎛ +

=

? ) ( '

, f x =

Contoh

Tentukan turunan fungsi

f ( x ) = (sin x )

^4x

)) ln(sin(

4 )

ln(sin )

(

ln f x = x

^4x

= x x

Jawab

))) ln(sin(

4 ( ))

(

(ln f x D x x

D

_x

=

_x

x x

x x x

x x x

f x

f cos 4 ln(sin( )) 4 cot

sin )) 4

ln(sin(

) 4 (

) (

' = + = +

x

x

x x

x x

f ' ( ) = ( 4 ln(sin( )) + 4 cot )(sin )

⁴

Ubah bentuk fungsi pangkat fungsi menjadi perkalian fungsi dengan menggunakan fungsi logaritma asli

Turunkan kedua ruas

B. Selesaikan integral tak tentu berikut 4

2x 1 dx

∫

+ ^{4 2}

2 5 x

x +x dx

∫

+ +

( )

2

x x 2 dx

∫

ln

∫

^ln _x^{3x dx}

2

∫

^tan(ln_x ^{x) dx}

x

x dx

3 2 +1

∫

(x+ )e^{x + x}dx

∫

³

2 6

( )

e^−x − e^−x dx

∫

^{sec2 2}

(cos ) x e

^{sin x}

dx

∫

e ^{2 ln x} dx

∫

∫

^x2e2x3dx

∫ _e ^e

^x

₊ ^dx

x

3

2

∫ ₋ ^e _e

^x

^dx

x

2 3 3

) 2

1 (

1.

2.

3.

4.

5.

6.

7.

8.

9.

10.

11.

12.

13.

C. Selesaikan integral tentu berikut 3

11 2

4

∫

− _x ^dx

( )

1 1 1

4

x x dx

∫ +

e

e dx

x x +

−

∫ ₃ 4

3 ln ln

( )

e^x 3 4e^x dx 0

5

∫ − ln

e^{2x 3}dx

0

1 +

∫

dx e ^x

∫

⁻

2 ln

0 3

e

x ^x dx

3

1 2 2

∫

∫

⁻

2

0

4 ²

dx xe

^x

∫

2

)

2

(ln

e

e

x x

dx

1.

2.

3.

4.

5.

6.

7.

8.

9.

x

x

x

⁻

→

1 1

lim

1

( )

^x

x

x

¹

0

1 sin 2 lim +

→

lim cos x

x

→∞ x

⎡

⎣⎢

⎤

⎦⎥

2 ²

(

^x

)

^x

x

e

^{2 ln1}

0

1

lim

₊

−

→

( )

^x

x

1 x

^{2 ln1}

lim +

∞

→

( )

^x

x

ln x

¹

lim

→∞

(

^{x x}

)

^x

x

1

5 3

lim +

∞

→

x

lim

x

x

→∞

x

+ +

⎛

⎝ ⎜ ⎞

⎠ ⎟

1 2

D. Hitung limit berikut :

1.

2.

3.

4.

5.

6.

7.

8.

8.5 Fungsi Eksponen Umum

Fungsi , a > 0 disebut fungsi eksponen umum

Untuk a > 0 dan x ∈ R, definisikan Turunan dan integral

Jika u = u(x), maka

Dari sini diperoleh : :

a

x

x f ( ) =

a

^x

= e

^{x ln a}

a a

a e

e D a

D

_x

(

^x

) =

_x

(

^xlna

) =

^xlna

ln =

^x

ln a u

a u

a e

e D a

D

_x

(

^u

) =

_x

(

^ulna

) =

^ulna

ln . ' =

^u

' ln

∫ ^a

^x

^{dx =} _ln ¹ _a ^a

^x

^{+ C}

Sifat–sifat fungsi eksponen umum

Untuk a > 0, b > 0, x, y bilangan riil berlaku

y x y

x

a a

a =

⁺

y x y

x

a a

a

₋

=

xy y

x

a

a ) = (

x x x

b a b

a ⎟ =

⎠

⎜ ⎞

⎝

⎛

1.

2.

3.

4.

( ab )

^x

= a

^x

b

^x

5.

∫ ⁴

^x2

^{. xdx}

C

du ^u C ^x

u = + = +

∫

⁴ _{2 2}¹ _ln⁴ _{4 2}⁴_ln4

2

x

x

x

f ( ) = 3

^{2 +1}

+ 2

^sin2

2 ln 2

cos 2

. 2 3

ln 3

. 2 )

(

' x

^{2 1 sin2}

x

f =

^x+

+

^x

Contoh

1. Hitung turunan pertama dari

Jawab :

2. Hitung

Jawab :

du dx

xdx du

x

u =

²

→ = 2 → =

₂¹_x

Misal

∫

^4x2.xdx =

Grafik fungsi eksponen umum

0 ,

)

( x = a a >

f

^x

) , ( −∞ ∞ Df =

a.

b.

f ' ( x ) = a

^x

ln a

⎩⎨

⎧

>

>

<

<

= <

1 ,

0 ln

1 0

, 0 ln

a a

a

a a

a

x x

f monoton naik jika a > 1

monoton turun jika 0 < a < 1

f

x

a x D

a x

f '' ( ) = (ln )

²

> 0 ∀ ∈

c.

Grafik f selalu cekung keatas d. f(0) = 1

Diketahui

1 ,

)

(x = a a >

f ^x

1 0

, )

(x = a <a <

f ^x

8.6 Fungsi Logaritma Umum

Karena fungsi eksponen umum monoton murni maka ada Inversnya. Invers dari fungsi eksponen umum disebut fungsi Logaritma Umum

( logaritma dengan bilangan pokok a ), notasi , sehingga berlaku :

Dari hubungan ini, didapat

Sehingga

Jika u=u(x), maka

a

log x

a

y

x =

⇔

x y =

^a

log

a x x

a y x

a y

a

x

^{y a}

ln log ln

ln ln ln

ln

ln = = ⇒ = ⇒ =

a x

a D x

x

D_x ^a _x

ln ) 1

ln (ln )

log

( = =

a u

u a

D u u

D_x ^a _x

ln ) '

ln (ln )

log

( = =

Contoh Tentukan turunan pertama dari

) 1 log(

)

( x =

³

x

²

+ f

1 ) log( 1

)

(

⁴

−

= +

x x x

f

1.

2.

Jawab :

1. ln3

) 1 ) ln(

1 log(

) (

2 2

3 +

= +

= x

x x

f

ln 3

1 1 ) 2

(

'

₂

= + x x x

f

2.

ln 4

) ) ln(

1 log( 1

)

(

¹

4 −1

+

− =

= +

^x

x

x x x

f ( ) ^{1 )}

( 1 1

4 ln ) 1

( '

11

−

= +

−

+

x

Dx x x

f

xx

)2

1 (

) 1 (

1 1

1 4

ln 1

− +

−

− +

= −

x x x

x x

2

1 −

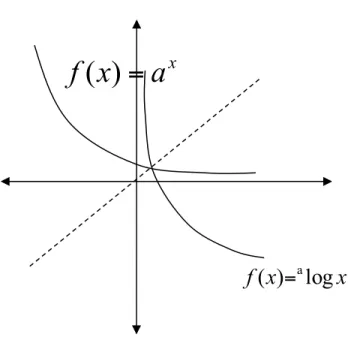
Grafik fungsi logaritma umum

Untuk a > 1

a

x

x f ( ) =

Untuk 0 < a < 1

ax

x f ( ) =

Grafik fungsi logaritma umum diperoleh dengan mencerminkan grafik fungsi eksponen umum terhadap garis y=x

x x

f ( )=^alog

x x

f( )=^alog

y = 3

^2x4−4x

( ⁹ )

log

²

10

+

= x

y

x 2 ^x

²

dx

∫

10

^{5 1x−}

dx

∫

Soal Latihan

A. Tentukan dari

y '

1.

2.

2 )

3

log(

= + y xy

3.

x

B. Hitung 1.

2.

8.7 Fungsi Invers Trigonometri

Fungsi trigonometri adalah fungsi yang periodik sehingga tidak satu-satu, jika daerah asalnya dibatasi, fungsi trigonometri bisa dibuat menjadi satu- satu sehingga mempunyai invers.

a. Invers fungsi sinus

Diketahui f(x) = sinx , ⁻₂^π

≤ x ≤

^π₂

Karena pada f(x)=sinx monoton murni maka inversnya ada.

Invers dari fungsi sinus disebut arcus sinus, notasi arcsin(x),atau sin⁻¹(x)

2 2π

≤ ≤

π

−

x

Sehingga berlaku

y x

x

y = sin

⁻¹

⇔ = sin

2π

−

π2

Turunan

Dari hubungan y =sin⁻¹ x⇔ x =siny

y dy

dx dx

dy

cos 1 /

1 =

=

2

, 2

1

1≤ ≤ ^π ≤ ≤ ^π

− x ⁻ y

dan rumus turunan fungsi invers diperoleh

1

| 1 |,

1 sin

1 1

2

2 <

−

=

−

= x

x y

2 1

1 ) 1

(sin x x

D_x

= −

atau −

Jika u=u(x)

2 1

1 ) '

(sin u

u u D_x

−

− =

Dari rumus turunan diperoleh

∫

^{dx 2 = sin−1 x +C}

b. Invers fungsi cosinus

Fungsi f(x) = cosx ,0 ≤ x ≤π

π

x x

f( ) = cos

monoton murni(selalu monoton turun), sehingga mempunyai invers

Definisi : Invers fungsi cosx disebut arcuscosx, notasi arc cosx atau cos⁻¹(x)

Berlaku hubungan

y x

x

y = cos

⁻¹

⇔ = cos

Turunan Dari

y = cos

⁻¹

x ⇔ x = cos y

dy 1 − 1

=

=

π

≤

≤

≤

≤

− 1 x 1 , 0 y ,

1

| 1 |,

1 − <

− =

= x

diperoleh

atau

2 1

1 ) 1

(cos x x

D_x

−

= −

−

2 1

1 ) '

(cos u

u u D_x

−

= −

Jika u= u(x) −

Dari rumus turunan diatas diperoleh

∫

₁^dx_{− x}^{2 =−cos−1 x+C}

Contoh

−

( )) = (sin

¹

x

²

D

_x ^{( )}

) ( 1

1 ₂

2

2 D x

x ^x

− 1 ⁴

2 x x

−

=

−

(tan )) = (cos

¹

x

D

_x ^{(tan )}

) (tan 1

1

2 D x

x ^x

−

−

x x

2 2

tan 1

sec

−

= −

Contoh Hitung

∫ _{4 −} ¹ _x

²

^dx

Jawab :

Gunakan rumus

∫

₁₋¹_u^{2 du = sin−1(u)+C}

∫

−

= dx

x ) 1 4

( 4

1

∫

₄¹_{− x}^{2 dx} 2

∫

−

= dx

x ₂ 2) ( 1 (

1 2

1

Misal

x du dx dx du

u 2

2

²

1

→ =

=

→

=

∫

₄¹_{− x}^{2 dx = 1}₂

∫

₍₁²_−u^{2 du = sin−1 u +C}

^{= sin}

⁻¹

⁽

^2x

^{) + C}

c. Invers fungsi tangen

Fungsi f(x) = tanx,

2 2π

≤ ≤

π

−

x

2π

− π2

f(x)=tanx

Monoton murni (selalu naik) sehingga mempunyai invers.

Definisi Invers dari tan x disebut fungsi arcus tanx, notasi arc tanx atau

tan

⁻¹

( x )

Berlaku hubungan

y x

x

y = tan

⁻¹

⇔ = tan

y x

x

y = tan

⁻¹

⇔ = tan

dy 1 1

=

=

Turunan Dari

2

,

⁻2^π

< y <

^π

dan turunan fungsi invers diperoleh

1

1 =

=

2 1

1 ) 1

(tan x x

D_x

= +

−

2 1

1 ) '

(tan u

u u D_x

= +

−

∫

₁₊^dx_x^{2 = tan−1 x + C}

atau

Jika u=u(x)

d. Invers fungsi cotangen

Fungsi f(x)= cot x

, 0 < x < π

π

f(x)=cotx

selalu monoton turun(monoton murni) sehingga mempunyai invers

Definisi Invers dari fungsi cot x disebut Arcus cotx, notasi arc cotx atau cot⁻¹ x

Berlaku hubungan

y x

x

y = cot

⁻¹

⇔ = cot

Turunan

y dy

dx dx

dy

csc

2

1 /

1 = −

=

_{2 2}

1 1 cot

1

1

x

y +

= − +

= −

atau 2 1

1 ) 1

(cot x x

D_x

+

= −

−

∫

_{4 +}^dx_x²

∫ _{1 +} ^dx _x

²

^{= − cot}

⁻¹

^{x + C}

2 1

1 ) '

(cot u

u u D_x

+

= − Jika u=u(x) −

Contoh

) 1 (

(tan

⁻¹

x

²

+

D

_x ( 1)

) 1 (

1

1 ₂

2

2 +

+

= + Dx x

x

1 (

²

1 )

²

2 +

= +

x x

) (sin (cot

¹

x

D

_x ^{− (sin )}

) (sin 1

1

2 Dx x

+ x

= −

x x sin2

1

cos +

= −

Contoh Hitung a.

b.

∫

^dx

∫ ∫

+

+ = dx

dx x

x )

1 4 ( 4

1 4

1

2 2

Jawab

a.

∫

+

= dx

x ₂ 2) ( 1

1 4

1

du dx

dx x du

u 2

2

²

1

→ =

=

→

=

∫ _{4 +} ¹ _x

²

^{dx =} ₄ ¹ ∫ _{1 +} ² _u

²

^{du = 1} ₂ ^tan

⁻¹

^{u + C}

x C +

=

⁻

)

( 2 2 tan

1

₁

Gunakan rumus

∫

₁₊¹_u^{2 du = tan−1(u)+C}

b.

Gunakan rumus

∫

₁₊¹_u^{2 du = tan−1(u)+C}

∫

∫

_x² ₊^dx_{2x + 4} ⁼ _{(x +1}¹₎² _{+ 3} ^dx

∫

₊

+

= dx

x )

3 ) 1 1 (

( 3

1

2

∫

⎟⎟ ⎠

⎞

⎜⎜ ⎝

⎛ +

+

= dx

x

²

3 ) 1 1 (

1 3

1

du dx

dx x du

u 3

3 1 3

1 → = → =

= + Misal

∫

∫ _x

²

₊ ^dx _{2 x + 4} ⁼ ₃ ¹ _{1 +} ³ _u

²

^{du = 1} ₃ ^tan

⁻¹

^{u + C}

x C

⎟⎟ +

⎞

⎜⎜

⎛ +

= ⁻ 1

1 tan ₁

e. Invers fungsi secan

Diberikan f(x) = sec x

, 2

0

, ≤ x ≤

π

x ≠ ^π

,

2

0 , 0 tan

sec )

(

' x = x x > ≤ x ≤ π x ≠

^π

f

f(x) = sec x monoton murni Ada inversnya

Definisi Invers dari fungsi sec x disebut arcus secx, notasi arc secx atau sec⁻¹ x

Sehingga

y x

x

y = sec

⁻¹

⇔ = sec

Turunan

Dari

y = sec

⁻¹

x ⇔ x = sec y

y 1 x

cos = y = cos

⁻¹

( )

¹x

( )

x

x

^{1 1}

1

cos

sec

⁻

=

⁻

Sehingga

( )

x x

x

x D

D (sec

⁻¹

) = (cos

^{−1 1} ₂

1 2

1 )

( 1

1

x

x

−

−

= −

1

|

|

2

2

−

= x x x

1

|

|

1

2 −

= x x

Jika u = u(x)

1

|

| ) '

(sec 2

1

−

− =

u u u u

D_x

∫ _{x x} ¹

²

₁ ^{dx = sec}

⁻¹

^{| x | + c}

e. Invers fungsi cosecan

Diberikan f(x) = csc x , ⁻₂^π ≤ x ≤ ^π₂ ,x ≠ 0

0 ,

, 0 cot

csc )

(

' x = − x x <

⁻₂

≤ x ≤

₂

x ≠

f

^{π π}

f(x) = sec x monoton murni Ada inversnya

Definisi Invers dari fungsi csc x disebut arcus csc x, notasi arc cscx atau csc⁻¹ x

Sehingga

y x

x

y = csc

⁻¹

⇔ = csc

Turunan

Dari

y = csc

⁻¹

x ⇔ x = csc y

y 1 x

sin = y = sin

⁻¹

( )

¹x

( )

x

x

^{1 1}

1

sin

csc

⁻

=

⁻

Sehingga

( )

x x

x

x D

D (csc

⁻¹

) = (sin

^{−1 1} ₂

1 2

1 )

( 1

1

x

x

−

= −

1

|

|

2

2

−

= −

x x

x

1

|

|

1

2 −

= −

x x

Jika u = u(x)

1

|

| ) '

(sec 2

1

−

= −

−

u u u u

D_x

∫ _{x x} ¹

²

₁ ^{dx = − csc}

⁻¹

^{| x | + c}

Contoh

A. Hitung turunan pertama dari

) ( sec )

( x

¹

x

²

f =

⁻

1 ) 2

1 ( )

(

|

| ) 1 (

'

2 4

2 2

2

2

= −

−

= x x

x x x Dx

x x

f

1

2

4 −

= x x a.

b.

Jawab a.

) (tan sec

)

( x

¹

x

f =

⁻

1 x tan

| x tan

|

x ) sec

x (tan 1 Dx

) x (tan

| x tan

| ) 1 x ('

f

2

2

2

−

=

−

b.

=

B. Hitung

∫

_{x x}¹² _{− 4} ^dx

∫

∫

−

=

−

x dx x

x dx

x 1)

( 4 4

1 4

1

2

2

∫

⎟ −

⎠

⎜ ⎞

⎝

⎛

= dx

x x 1

2 1 2

1

2

Jawab

Misal

x du dx dx du

u 2

2

²

1

→ =

=

→

=

∫ ∫

∫

_{x x}¹² _{− 4} ^{dx =} ₂¹ _{2u u}¹² ₋₁ ^{2du =} ₂¹ _{u u}¹² ₋₁ ^du

x 1

1

Soal Latihan

2 1

) (sin x y =

⁻

) ( tan

¹

e

^x

y =

⁻

x x

y = tan

⁻¹

ln

e

t

t

f ( ) =

^sec−1

) 3 ( cot

¹

2

x

x

y =

⁻

) 1

(

tan

¹

x x

²

y =

⁻

− +

A. Tentukan turunan pertama fungsi berikut, sederhanakan jika mungkin 1.

2.

3.

4.

5.

6.

B. Hitung

∫ _{9 x}

²

^dx _{+ 16}

∫

_{4x x}^dx2 ₋₁₆

∫ ₂ ^dx _{− 5 x}

²

∫ ₋

2 − / 1

0 2

1

1

sin dx x

x

∫ _e ^e

^x

₊ ^dx

x 2

1

∫ ^e _{− e}

^x

^dx

x 4 2

1

∫

_x[4+^dx_{(ln x)}²_]

1.

2.

3.

4.

5.

6.

7.

8.8 Fungsi Hiperbolik

Definisi

a. Fungsi kosinus hiperbolik :

cosh 2 )

(

x

x e

x e x

f + ⁻

=

= b. Fungsi sinus hiperbolik :

sinh 2 )

(

x

x

e

x e x

f −

⁻

=

=

c. Fungsi tangen hiperbolik : _{x x}

x x

e e

e e

x x x

x

f ⁻₋

+

= −

=

= cosh

tanh sinh )

(

x x

x x

e e

e e

x x x

x

f ₋⁻

−

= +

=

= sinh

coth cosh )

(

x

x e

e x x

h x

f ₋

= −

=

= 2

cosh sec 1

) (

x

x e

e x x

h x

f −

= +

=

= 2

sinh csc 1

) (

d. Fungsi cotangen hiperbolik : e. Fungsi secan hiperbolik : f. Fungsi cosecan hiperbolik :

1 sinh

cosh

²

x −

²

x = x

h

x

²

2

sec

tanh

1 − =

x h

x

²

2

1 csc

coth − =

Persamaan identitas pada fungsi hiperbolik

e

x

x x + sinh = cosh

1.

2.

cosh x − sinh x = e

^{−x 3.}

4.

5.

Turunan

e x e

e D e

x D

x x

x x

x

x cosh

2 ) 2

(sinh + =

⎟⎟ =

⎠

⎞

⎜⎜⎝

⎛ −

=

−

−

e x e

e D e

x D

x x

x x

x

x sinh

2 ) 2

(cosh − =

⎟⎟ =

⎠

⎞

⎜⎜⎝

⎛ +

=

−

−

∫ ^{sinh x dx = cosh x + C}

∫ ^{cosh x dx = sinh x + C}

cosh ) (sinh )

(tanh

x D x

x

D_x = _x h x

x x

x

x ₂

2 2

2 2

cosh sec 1 cosh

sinh

cosh − = =

= sinh )

(cosh )

(coth

x D x

x

D_x = _x

x

x x

x

x x

2

2 2

2

2 2

sinh

) sinh (cosh

sinh

cosh

sinh − −

− =

=

x x h

2

2 csc

sinh

1 = −

= − cosh )

( 1 )

(sechx D x

D_x = _x hx x

x

x sec tanh cosh

sinh

2 = −

= −

sinh ) ( 1 )

(cschx D x

D_x = _x hx x

x

x csc coth sinh

cosh

2 = −

= −